168 CHAPTER 6. FIXED POINT THEOREMS

Lemma 6.5.3 If f is upper semicontinuous on some set K and g is continuous anddefined on f (K) , then g ◦f is also upper semicontinuous.

Proof: Let xn→ x in K. Let U ⊇ g ◦f (x) . Is g ◦f (xn) ∈U for all n large enough?We have f (x) ∈ g−1 (U) , an open set. Therefore, if n is large enough, f (xn) ∈ g−1 (U).It follows that for large enough n, g ◦f (xn) ∈U and so g ◦f is upper semicontinuous onK. ■

The next theorem is an application of the Brouwer fixed point theorem. First define anp simplex, denoted by [x0, · · · ,xp], to be the convex hull of the p+1 points,

{x0, · · · ,xp

}where {xi−x0}p

i=1 are independent. Thus

[x0, · · · ,xp]≡

{p

∑i=1

tixi :p

∑i=1

ti = 1, ti ≥ 0

}.

If p ≤ 2, the simplex is a triangle, line segment, or point. If p ≤ 3, it is a tetrahedron,triangle, line segment or point. A collection of simplices is a tiling of Rp if Rp is containedin their union and if S1,S2 are two simplices in the tiling, with

S j =[x j

0, · · · ,xjp

],

thenS1∩S2 =

[xk0 , · · · ,xkr

]where {

xk0 , · · · ,xkr

}⊆{x1

0, · · · ,x1p}∩{x2

0, · · · ,x2p}

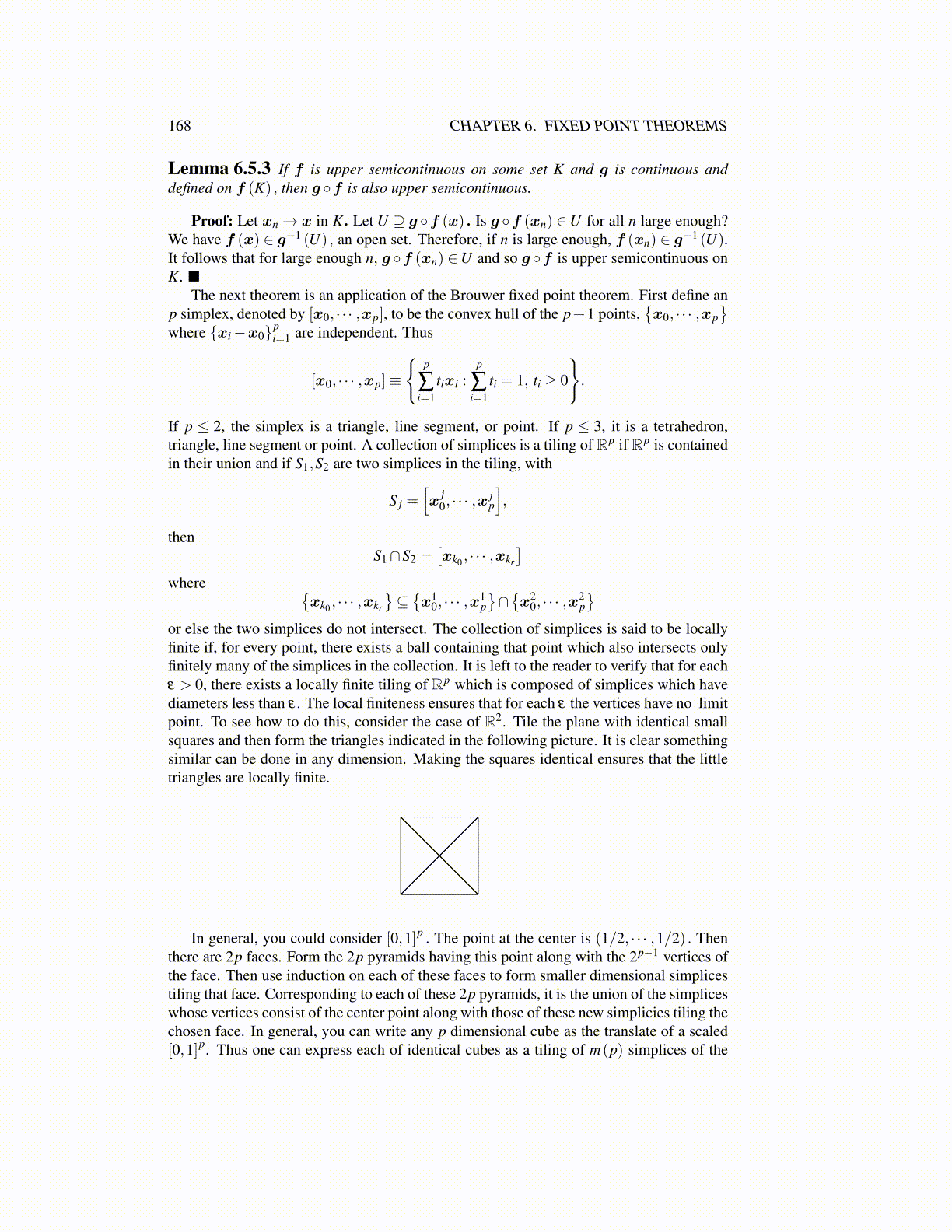
or else the two simplices do not intersect. The collection of simplices is said to be locallyfinite if, for every point, there exists a ball containing that point which also intersects onlyfinitely many of the simplices in the collection. It is left to the reader to verify that for eachε > 0, there exists a locally finite tiling of Rp which is composed of simplices which havediameters less than ε . The local finiteness ensures that for each ε the vertices have no limitpoint. To see how to do this, consider the case of R2. Tile the plane with identical smallsquares and then form the triangles indicated in the following picture. It is clear somethingsimilar can be done in any dimension. Making the squares identical ensures that the littletriangles are locally finite.

In general, you could consider [0,1]p . The point at the center is (1/2, · · · ,1/2) . Thenthere are 2p faces. Form the 2p pyramids having this point along with the 2p−1 vertices ofthe face. Then use induction on each of these faces to form smaller dimensional simplicestiling that face. Corresponding to each of these 2p pyramids, it is the union of the simpliceswhose vertices consist of the center point along with those of these new simplicies tiling thechosen face. In general, you can write any p dimensional cube as the translate of a scaled[0,1]p. Thus one can express each of identical cubes as a tiling of m(p) simplices of the