246 CHAPTER 9. MEASURES AND MEASURABLE FUNCTIONS

Definition 9.5.2 (µ⌊S)(A) ≡ µ(S∩A) for all A ⊆ Ω. Thus µ⌊S is the name of anew outer measure, called µ restricted to S.

The next lemma indicates that the property of measurability is not lost by consideringthis restricted measure.

Lemma 9.5.3 If A is µ measurable, then for any S, A is µ⌊S measurable.

Proof: Suppose A is µ measurable. It is desired to to show that for all T ⊆Ω,

(µ⌊S)(T ) = (µ⌊S)(T ∩A)+(µ⌊S)(T \A).

Thus it is desired to show

µ(S∩T ) = µ(T ∩A∩S)+µ(T ∩S∩AC). (9.7)

But 9.7 holds because A is µ measurable. Apply Definition 9.5.1 to S∩T instead of S. ■If A is µ⌊S measurable, it does not follow that A is µ measurable. Indeed, if you believe

in the existence of non measurable sets which is discussed later, you could let A = S forsuch a µ non measurable set and verify that S is µ⌊S measurable.

The next theorem is the main result on outer measures which shows that starting withan outer measure you can obtain a measure.

Theorem 9.5.4 Let Ω be a set and let µ be an outer measure on P (Ω). The col-lection of µ measurable sets S , forms a σ algebra and

If Fi ∈S, Fi∩Fj = /0, then µ(∪∞i=1Fi) =

∞

∑i=1

µ(Fi). (9.8)

If · · ·Fn ⊆ Fn+1 ⊆ ·· · , then if F = ∪∞n=1Fn and Fn ∈S , it follows that

µ(F) = limn→∞

µ(Fn). (9.9)

If · · ·Fn ⊇ Fn+1 ⊇ ·· · , and if F = ∩∞n=1Fn for Fn ∈S then if µ(F1)< ∞,

µ(F) = limn→∞

µ(Fn). (9.10)

This measure space is also complete which means that if µ (F) = 0 for some F ∈S thenif G⊆ F, it follows G ∈S also.

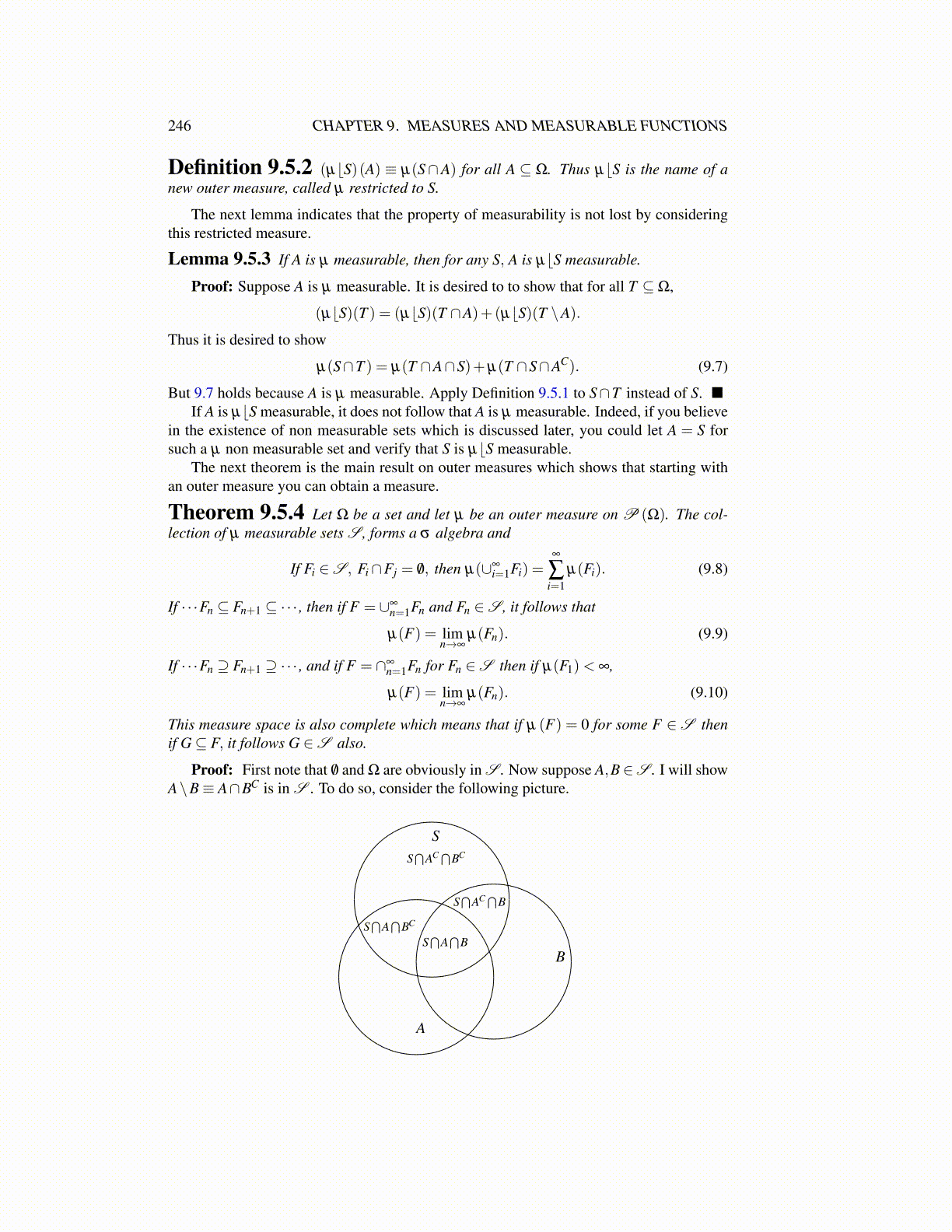
Proof: First note that /0 and Ω are obviously in S . Now suppose A,B∈S . I will showA\B≡ A∩BC is in S . To do so, consider the following picture.

S⋂

AC⋂BC

S⋂

AC⋂B

S⋂

A⋂

BS⋂

A⋂

BC

A

B

S