9.11. COMPLETION OF A MEASURE SPACE 261

Proof: Define the outer measure

λ (A)≡ inf{µ (E) : E ∈F ,E ⊇ A} , λ ( /0)≡ 0.

Denote by G the σ algebra of λ measurable sets. Then (Ω,G ,λ ) is complete by the generalCaratheodory procedure presented earlier.

I claim that λ = µ on F . If A ∈F ,

µ (A)≤ inf{µ (E) : E ∈F ,E ⊇ A} ≡ λ (A)≤ µ (A)

because A⊇ A. Thus, these are all equal in the above and λ = µ on F .Why is F ⊆ G ? Letting λ (S)< ∞, (There is nothing to prove if λ (S) = ∞.) let G ∈F

be such that G⊇ S and λ (S) = µ (G) . This is possible because

λ (S)≡ inf{µ (E) : E ⊇ S and E ∈F} .

Then if A ∈F ,

λ (S) ≤ λ (S∩A)+λ(S∩AC)≤ λ (G∩A)+λ

(G∩AC)

= µ (G∩A)+µ(G∩AC)= µ (G) = λ (S) .

Thus F ⊆ G .Finally suppose µ is σ finite. Let Ω = ∪∞

n=1Ωn where the Ωn are disjoint sets of F

and µ (Ωn) < ∞. If the Ωn are not disjoint, replace Ωn with Ωn \∪n−1k=1Ωk. Letting A ∈ G ,

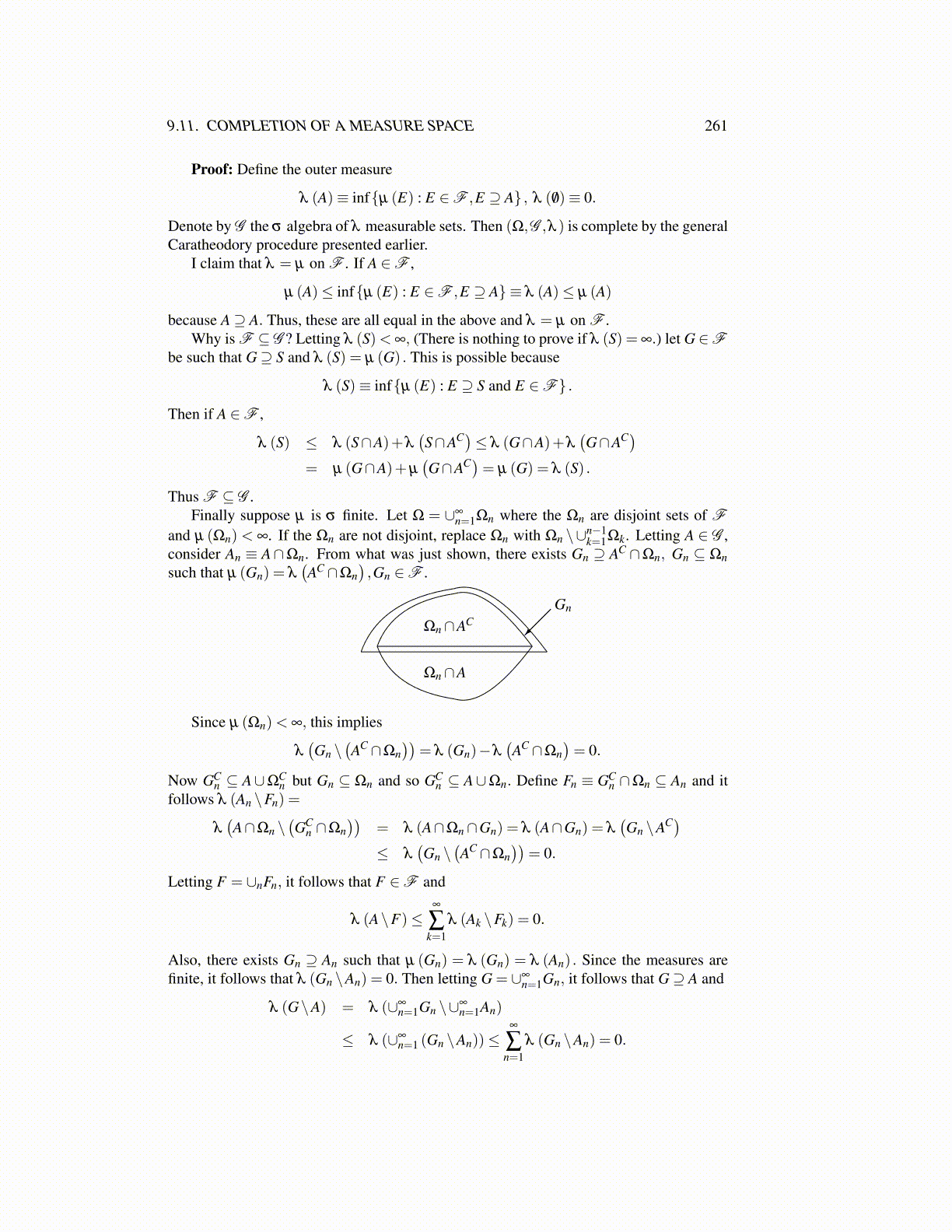
consider An ≡ A∩Ωn. From what was just shown, there exists Gn ⊇ AC ∩Ωn, Gn ⊆ Ωnsuch that µ (Gn) = λ

(AC ∩Ωn

),Gn ∈F .

Ωn∩AC

Ωn∩A

Gn

Since µ (Ωn)< ∞, this implies

λ(Gn \

(AC ∩Ωn

))= λ (Gn)−λ

(AC ∩Ωn

)= 0.

Now GCn ⊆ A∪ΩC

n but Gn ⊆ Ωn and so GCn ⊆ A∪Ωn. Define Fn ≡ GC

n ∩Ωn ⊆ An and itfollows λ (An \Fn) =

λ(A∩Ωn \

(GC

n ∩Ωn))

= λ (A∩Ωn∩Gn) = λ (A∩Gn) = λ(Gn \AC)

≤ λ(Gn \

(AC ∩Ωn

))= 0.

Letting F = ∪nFn, it follows that F ∈F and

λ (A\F)≤∞

∑k=1

λ (Ak \Fk) = 0.

Also, there exists Gn ⊇ An such that µ (Gn) = λ (Gn) = λ (An) . Since the measures arefinite, it follows that λ (Gn \An) = 0. Then letting G = ∪∞

n=1Gn, it follows that G⊇ A and

λ (G\A) = λ (∪∞n=1Gn \∪∞

n=1An)

≤ λ (∪∞n=1 (Gn \An))≤

∞

∑n=1

λ (Gn \An) = 0.