328 CHAPTER 11. REGULAR MEASURES

Lemma 11.6.4 If h∈C2(

B(0,R))

and h : B(0,R)→B(0,R), then h has a fixed point

x such that h(x) = x.

Proof: Suppose the lemma is not true. Then for all x, |x−h(x)| ̸= 0. Then defineg (x) = h(x)+ x−h(x)

|x−h(x)| t (x) where t (x) is nonnegative and is chosen such that g (x) ∈∂B(0,R) .

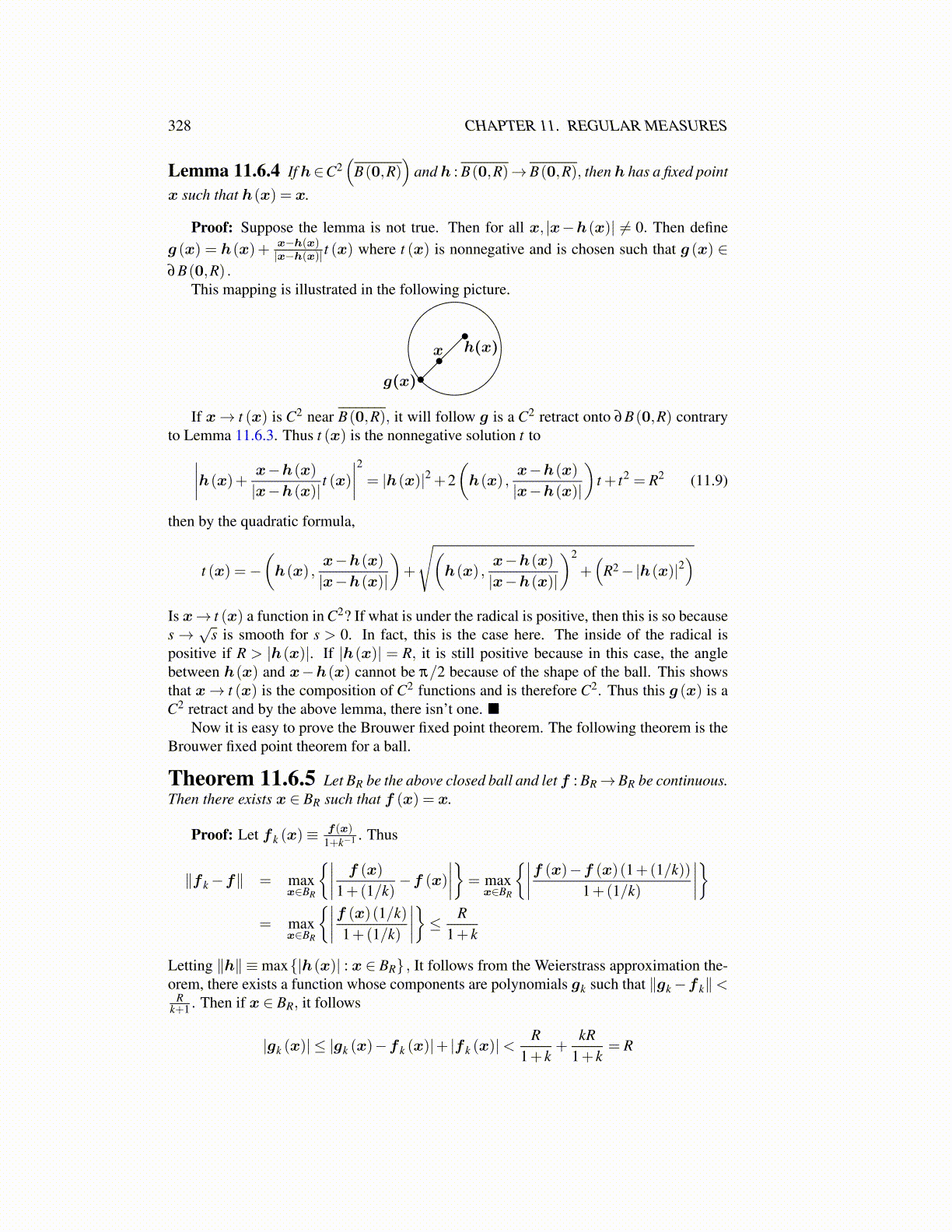
This mapping is illustrated in the following picture.

h(x)x

g(x)

If x→ t (x) is C2 near B(0,R), it will follow g is a C2 retract onto ∂B(0,R) contraryto Lemma 11.6.3. Thus t (x) is the nonnegative solution t to∣∣∣∣h(x)+ x−h(x)

|x−h(x)|t (x)

∣∣∣∣2 = |h(x)|2 +2(h(x) ,

x−h(x)|x−h(x)|

)t + t2 = R2 (11.9)

then by the quadratic formula,

t (x) =−(h(x) ,

x−h(x)|x−h(x)|

)+

√(h(x) ,

x−h(x)|x−h(x)|

)2

+(

R2−|h(x)|2)

Is x→ t (x) a function in C2? If what is under the radical is positive, then this is so becauses→√

s is smooth for s > 0. In fact, this is the case here. The inside of the radical ispositive if R > |h(x)|. If |h(x)| = R, it is still positive because in this case, the anglebetween h(x) and x−h(x) cannot be π/2 because of the shape of the ball. This showsthat x→ t (x) is the composition of C2 functions and is therefore C2. Thus this g (x) is aC2 retract and by the above lemma, there isn’t one. ■

Now it is easy to prove the Brouwer fixed point theorem. The following theorem is theBrouwer fixed point theorem for a ball.

Theorem 11.6.5 Let BR be the above closed ball and let f : BR→BR be continuous.Then there exists x ∈ BR such that f (x) = x.

Proof: Let f k (x)≡f(x)

1+k−1 . Thus

∥f k−f∥ = maxx∈BR

{∣∣∣∣ f (x)

1+(1/k)−f (x)

∣∣∣∣}= maxx∈BR

{∣∣∣∣f (x)−f (x)(1+(1/k))1+(1/k)

∣∣∣∣}= max

x∈BR

{∣∣∣∣f (x)(1/k)1+(1/k)

∣∣∣∣}≤ R1+ k

Letting ∥h∥ ≡max{|h(x)| : x ∈ BR} , It follows from the Weierstrass approximation the-orem, there exists a function whose components are polynomials gk such that ∥gk−f k∥<

Rk+1 . Then if x ∈ BR, it follows

|gk (x)| ≤ |gk (x)−f k (x)|+ |f k (x)|<R

1+ k+

kR1+ k

= R