12.1. BASIC INEQUALITIES AND PROPERTIES 361

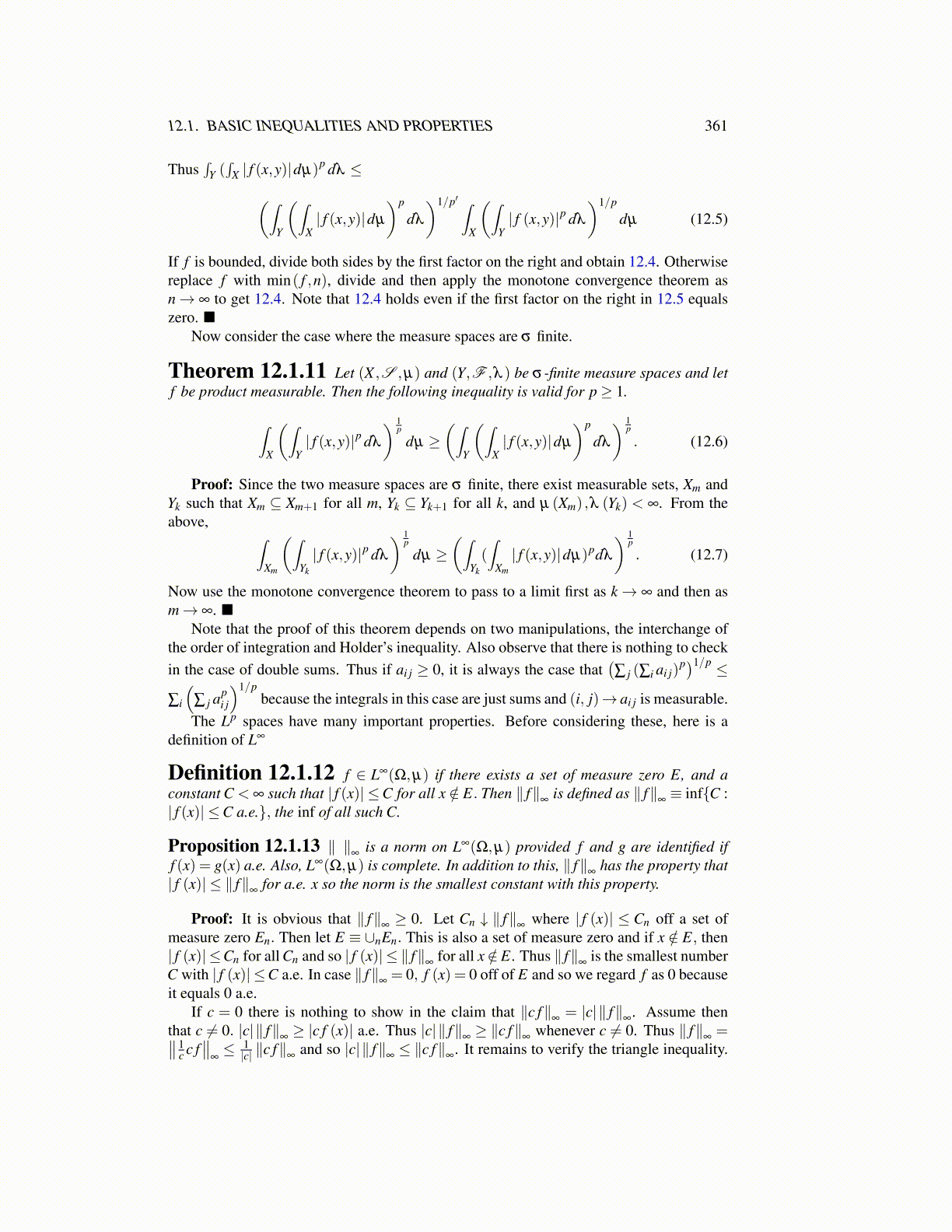
Thus∫

Y (∫

X | f (x,y)|dµ)p dλ ≤(∫Y

(∫X| f (x,y)|dµ

)p

dλ

)1/p′ ∫X

(∫Y| f (x,y)|p dλ

)1/p

dµ (12.5)

If f is bounded, divide both sides by the first factor on the right and obtain 12.4. Otherwisereplace f with min( f ,n), divide and then apply the monotone convergence theorem asn→ ∞ to get 12.4. Note that 12.4 holds even if the first factor on the right in 12.5 equalszero. ■

Now consider the case where the measure spaces are σ finite.

Theorem 12.1.11 Let (X ,S ,µ) and (Y,F ,λ ) be σ -finite measure spaces and letf be product measurable. Then the following inequality is valid for p≥ 1.

∫X

(∫Y| f (x,y)|p dλ

) 1p

dµ ≥(∫

Y

(∫X| f (x,y)|dµ

)p

dλ

) 1p

. (12.6)

Proof: Since the two measure spaces are σ finite, there exist measurable sets, Xm andYk such that Xm ⊆ Xm+1 for all m, Yk ⊆ Yk+1 for all k, and µ (Xm) ,λ (Yk) < ∞. From theabove, ∫

Xm

(∫Yk

| f (x,y)|p dλ

) 1p

dµ ≥(∫

Yk

(∫

Xm

| f (x,y)|dµ)pdλ

) 1p

. (12.7)

Now use the monotone convergence theorem to pass to a limit first as k→ ∞ and then asm→ ∞. ■

Note that the proof of this theorem depends on two manipulations, the interchange ofthe order of integration and Holder’s inequality. Also observe that there is nothing to checkin the case of double sums. Thus if ai j ≥ 0, it is always the case that

(∑ j (∑i ai j)

p)1/p ≤

∑i

(∑ j ap

i j

)1/pbecause the integrals in this case are just sums and (i, j)→ ai j is measurable.

The Lp spaces have many important properties. Before considering these, here is adefinition of L∞

Definition 12.1.12 f ∈ L∞(Ω,µ) if there exists a set of measure zero E, and aconstant C < ∞ such that | f (x)| ≤C for all x /∈ E. Then ∥ f∥

∞is defined as ∥ f∥

∞≡ inf{C :

| f (x)| ≤C a.e.}, the inf of all such C.

Proposition 12.1.13 ∥ ∥∞

is a norm on L∞(Ω,µ) provided f and g are identified iff (x) = g(x) a.e. Also, L∞(Ω,µ) is complete. In addition to this, ∥ f∥

∞has the property that

| f (x)| ≤ ∥ f∥∞

for a.e. x so the norm is the smallest constant with this property.

Proof: It is obvious that ∥ f∥∞≥ 0. Let Cn ↓ ∥ f∥

∞where | f (x)| ≤ Cn off a set of

measure zero En. Then let E ≡ ∪nEn. This is also a set of measure zero and if x /∈ E, then| f (x)| ≤Cn for all Cn and so | f (x)| ≤ ∥ f∥

∞for all x /∈ E. Thus ∥ f∥

∞is the smallest number

C with | f (x)| ≤C a.e. In case ∥ f∥∞= 0, f (x) = 0 off of E and so we regard f as 0 because

it equals 0 a.e.If c = 0 there is nothing to show in the claim that ∥c f∥

∞= |c|∥ f∥

∞. Assume then

that c ̸= 0. |c|∥ f∥∞≥ |c f (x)| a.e. Thus |c|∥ f∥

∞≥ ∥c f∥

∞whenever c ̸= 0. Thus ∥ f∥

∞=∥∥ 1

c c f∥∥

∞≤ 1|c| ∥c f∥

∞and so |c|∥ f∥

∞≤ ∥c f∥

∞. It remains to verify the triangle inequality.