15.1. SARD’S LEMMA AND APPROXIMATION 415
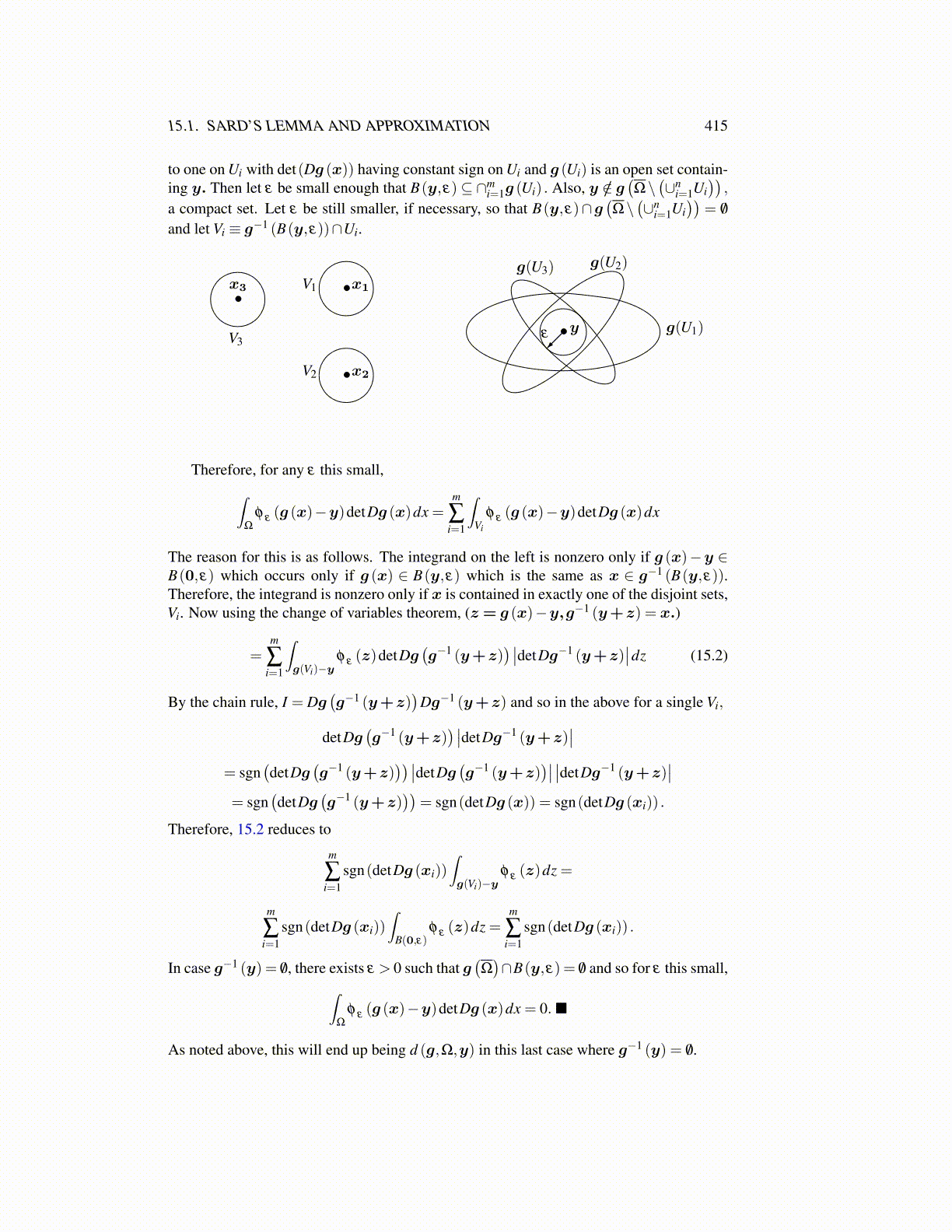
to one on Ui with det(Dg (x)) having constant sign on Ui and g (Ui) is an open set contain-ing y. Then let ε be small enough that B(y,ε)⊆ ∩m
i=1g (Ui) . Also, y /∈ g(Ω\(∪n
i=1Ui))
,
a compact set. Let ε be still smaller, if necessary, so that B(y,ε)∩g(Ω\(∪n
i=1Ui))
= /0and let Vi ≡ g−1 (B(y,ε))∩Ui.
g(U2)g(U3)
g(U1)•yε
•x1
•x2
•x3 V1
V2
V3
Therefore, for any ε this small,∫Ω
φ ε (g (x)−y)detDg (x)dx =m
∑i=1
∫Vi
φ ε (g (x)−y)detDg (x)dx
The reason for this is as follows. The integrand on the left is nonzero only if g (x)−y ∈B(0,ε) which occurs only if g (x) ∈ B(y,ε) which is the same as x ∈ g−1 (B(y,ε)).Therefore, the integrand is nonzero only if x is contained in exactly one of the disjoint sets,Vi. Now using the change of variables theorem, (z= g (x)−y,g−1 (y+z) = x.)
=m
∑i=1
∫g(Vi)−y
φ ε (z)detDg(g−1 (y+z)
)∣∣detDg−1 (y+z)∣∣dz (15.2)
By the chain rule, I = Dg(g−1 (y+z)
)Dg−1 (y+z) and so in the above for a single Vi,
detDg(g−1 (y+z)
)∣∣detDg−1 (y+z)∣∣
= sgn(detDg
(g−1 (y+z)
))∣∣detDg(g−1 (y+z)
)∣∣ ∣∣detDg−1 (y+z)∣∣
= sgn(detDg
(g−1 (y+z)
))= sgn(detDg (x)) = sgn(detDg (xi)) .
Therefore, 15.2 reduces to
m
∑i=1
sgn(detDg (xi))∫g(Vi)−y
φ ε (z)dz =
m
∑i=1
sgn(detDg (xi))∫
B(0,ε)φ ε (z)dz =
m
∑i=1
sgn(detDg (xi)) .
In case g−1 (y) = /0, there exists ε > 0 such that g(Ω)∩B(y,ε) = /0 and so for ε this small,∫
Ω
φ ε (g (x)−y)detDg (x)dx = 0.■
As noted above, this will end up being d (g,Ω,y) in this last case where g−1 (y) = /0.