58 CHAPTER 2. FUNCTIONS
In addition to this,
cos2x = cos2 x− sin2 x = 2cos2 x−1 = 1−2sin2 x (2.11)
Therefore, making use of the above identities,
cos(3x) = cos(2x+ x) = cos2xcosx− sin2xsinx
=(2cos2 x−1
)cosx−2cosxsin2 x
= 4cos3 x−3cosx (2.12)
For a systematic way to find cosine or sine of a multiple of x, see De Moivre’s theoremexplained in Problem 16 on Page 44.
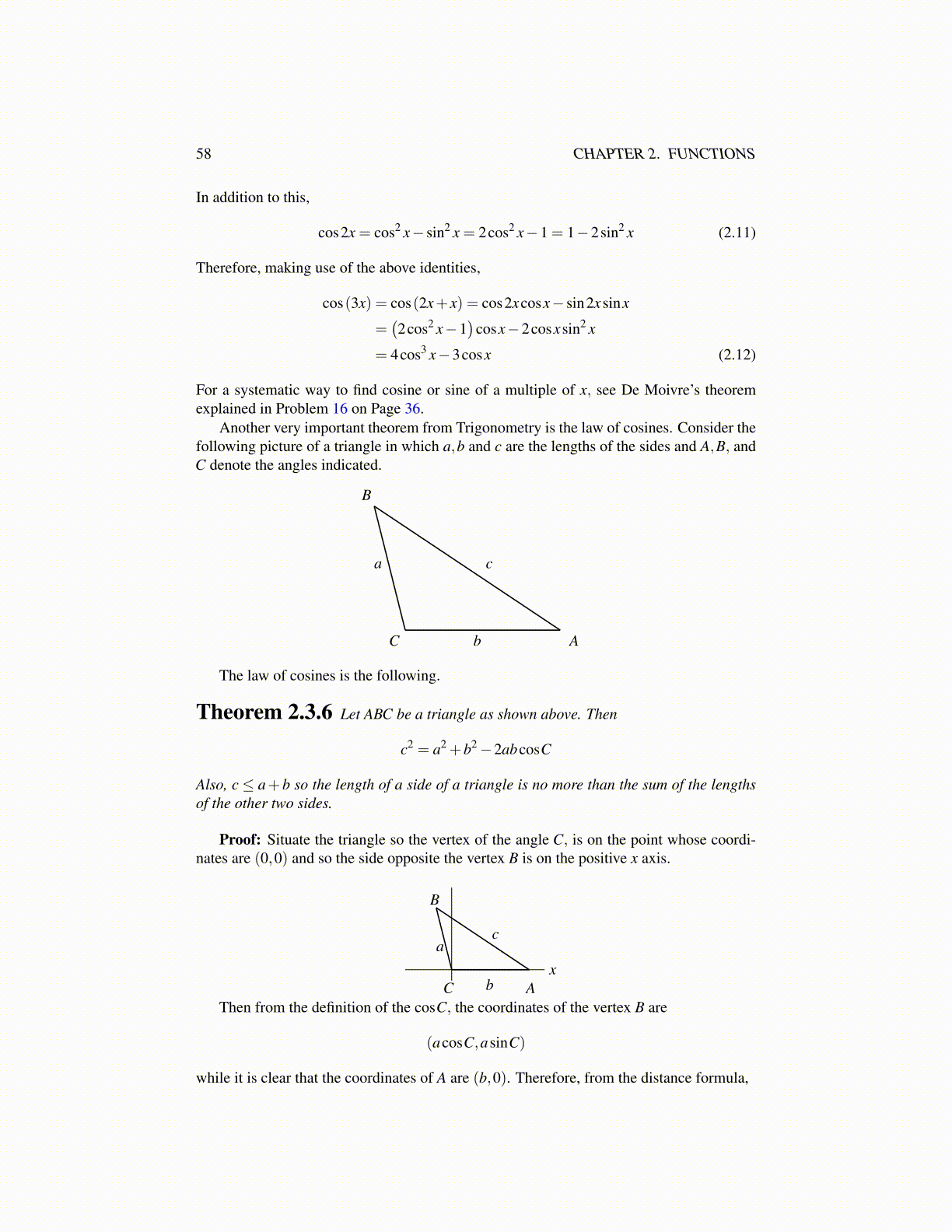
Another very important theorem from Trigonometry is the law of cosines. Consider thefollowing picture of a triangle in which a,b and c are the lengths of the sides and A,B, andC denote the angles indicated.
A
B
C
a
b
c
The law of cosines is the following.
Theorem 2.3.6 Let ABC be a triangle as shown above. Then
c2 = a2 +b2 −2abcosC
Also, c ≤ a+b so the length of a side of a triangle is no more than the sum of the lengthsof the other two sides.
Proof: Situate the triangle so the vertex of the angle C, is on the point whose coordi-nates are (0,0) and so the side opposite the vertex B is on the positive x axis.
A
B
C
a
b
c
x
Then from the definition of the cosC, the coordinates of the vertex B are
(acosC,asinC)
while it is clear that the coordinates of A are (b,0). Therefore, from the distance formula,