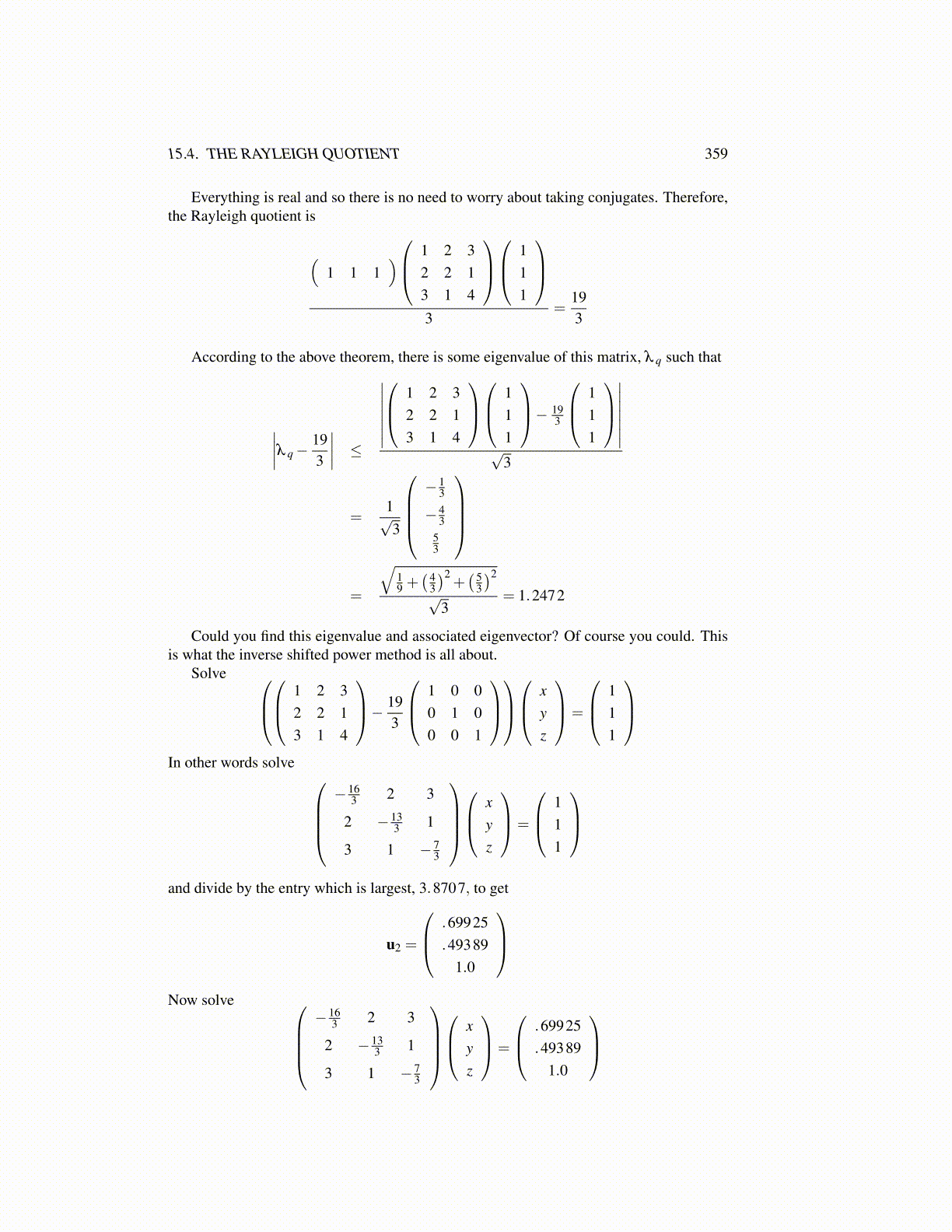
15.4. THE RAYLEIGH QUOTIENT 359

Everything is real and so there is no need to worry about taking conjugates. Therefore,the Rayleigh quotient is

(1 1 1

) 1 2 32 2 13 1 4

 1

11

3

=193

According to the above theorem, there is some eigenvalue of this matrix, λ q such that

∣∣∣∣λ q−193

∣∣∣∣ ≤∣∣∣∣∣∣∣ 1 2 3

2 2 13 1 4

 1

11

− 193

 111

∣∣∣∣∣∣∣

√3

=1√3

− 1

3

− 43

53

=

√19 +( 4

3

)2+( 5

3

)2

√3

= 1.2472

Could you find this eigenvalue and associated eigenvector? Of course you could. Thisis what the inverse shifted power method is all about.

Solve  1 2 3

2 2 13 1 4

− 193

 1 0 00 1 00 0 1

 x

yz

=

 111

In other words solve 

− 163 2 3

2 − 133 1

3 1 − 73

 x

yz

=

 111

and divide by the entry which is largest, 3.8707, to get

u2 =

 .69925.49389

1.0

Now solve 

− 163 2 3

2 − 133 1

3 1 − 73

 x

yz

=

 .69925.49389

1.0

