352 CHAPTER 19. THE RIEMANNN INTEGRAL ON Rn
For each y between 0 and 4, the variable x, goes from y to 4.∫ 4
y
(x2y+ yx
)dx =
883
y− 13
y4− 12
y3
Now ∫R
f dA =∫ 4
0
(883
y− 13
y4− 12
y3)
dy =672
5.
Here is a similar example.
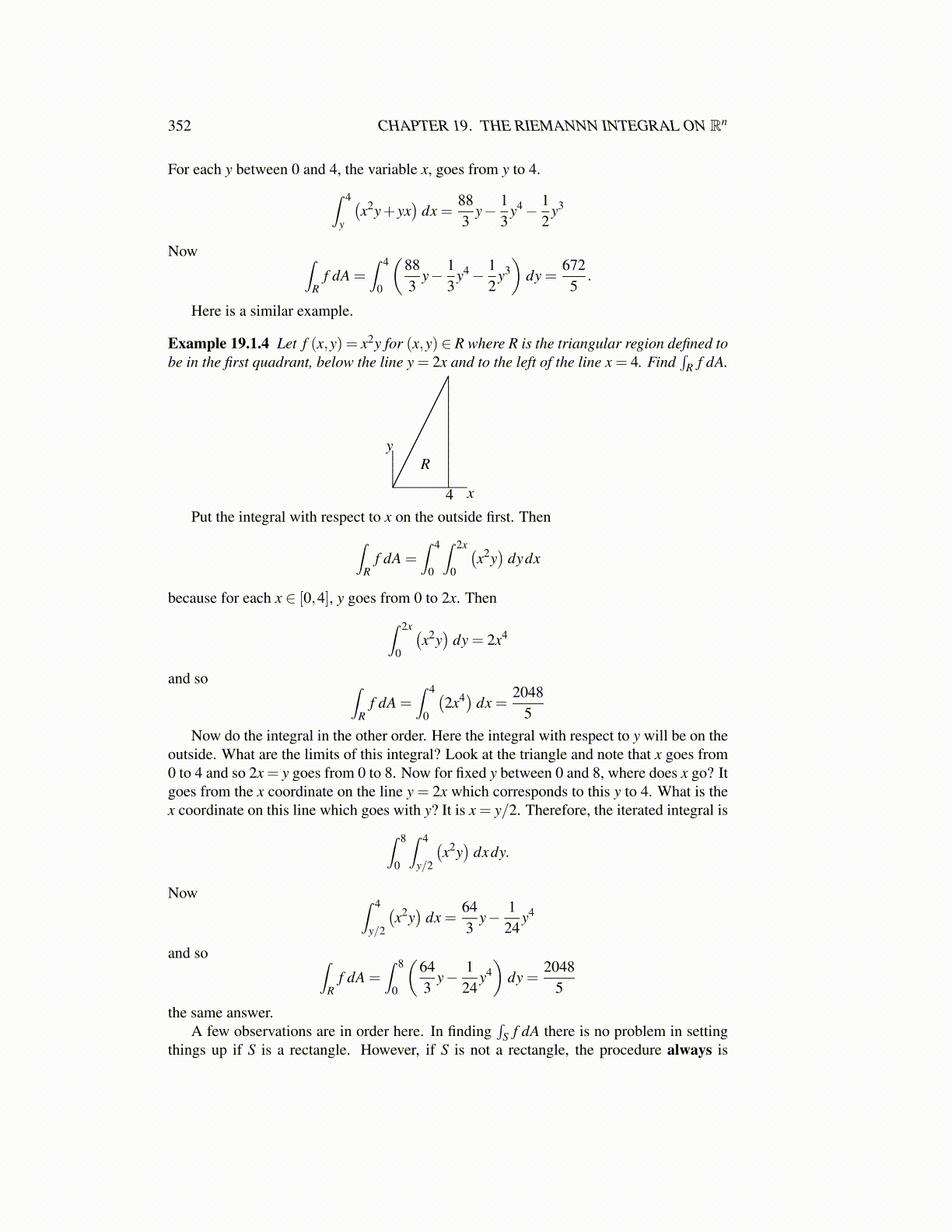
Example 19.1.4 Let f (x,y) = x2y for (x,y)∈ R where R is the triangular region defined tobe in the first quadrant, below the line y = 2x and to the left of the line x = 4. Find
∫R f dA.
x
y
4
R
Put the integral with respect to x on the outside first. Then∫R
f dA =∫ 4
0
∫ 2x
0
(x2y)
dydx
because for each x ∈ [0,4], y goes from 0 to 2x. Then∫ 2x
0
(x2y)
dy = 2x4
and so ∫R
f dA =∫ 4
0
(2x4) dx =
20485
Now do the integral in the other order. Here the integral with respect to y will be on theoutside. What are the limits of this integral? Look at the triangle and note that x goes from0 to 4 and so 2x = y goes from 0 to 8. Now for fixed y between 0 and 8, where does x go? Itgoes from the x coordinate on the line y = 2x which corresponds to this y to 4. What is thex coordinate on this line which goes with y? It is x = y/2. Therefore, the iterated integral is∫ 8
0
∫ 4
y/2
(x2y)
dxdy.
Now ∫ 4
y/2
(x2y)
dx =643
y− 124
y4
and so ∫R
f dA =∫ 8
0
(643
y− 124
y4)
dy =2048
5
the same answer.A few observations are in order here. In finding
∫S f dA there is no problem in setting
things up if S is a rectangle. However, if S is not a rectangle, the procedure always is