354 CHAPTER 19. THE RIEMANNN INTEGRAL ON Rn
In other words you integrate the density to get the mass. Now by letting ρ depend onposition, you can include the case where the material is not homogeneous. Here is anexample.
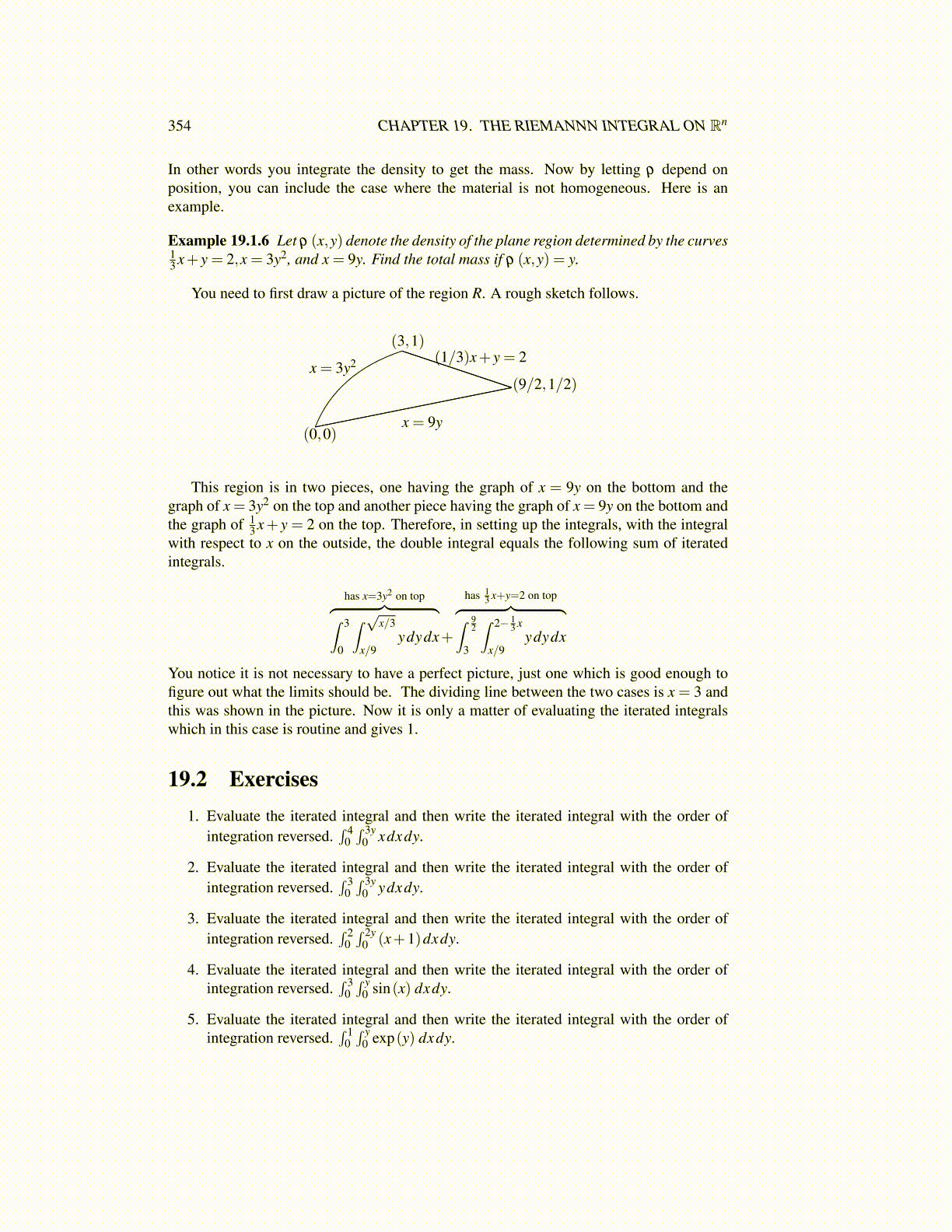
Example 19.1.6 Let ρ (x,y) denote the density of the plane region determined by the curves13 x+ y = 2,x = 3y2, and x = 9y. Find the total mass if ρ (x,y) = y.
You need to first draw a picture of the region R. A rough sketch follows.
(3,1)
(9/2,1/2)
(0,0)
x = 3y2 (1/3)x+ y = 2
x = 9y
This region is in two pieces, one having the graph of x = 9y on the bottom and thegraph of x = 3y2 on the top and another piece having the graph of x = 9y on the bottom andthe graph of 1
3 x+ y = 2 on the top. Therefore, in setting up the integrals, with the integralwith respect to x on the outside, the double integral equals the following sum of iteratedintegrals.
has x=3y2 on top︷ ︸︸ ︷∫ 3
0
∫ √x/3
x/9ydydx+
has 13 x+y=2 on top︷ ︸︸ ︷∫ 9
2
3
∫ 2− 13 x
x/9ydydx
You notice it is not necessary to have a perfect picture, just one which is good enough tofigure out what the limits should be. The dividing line between the two cases is x = 3 andthis was shown in the picture. Now it is only a matter of evaluating the iterated integralswhich in this case is routine and gives 1.
19.2 Exercises1. Evaluate the iterated integral and then write the iterated integral with the order of
integration reversed.∫ 4
0∫ 3y
0 xdxdy.
2. Evaluate the iterated integral and then write the iterated integral with the order ofintegration reversed.
∫ 30∫ 3y
0 ydxdy.
3. Evaluate the iterated integral and then write the iterated integral with the order ofintegration reversed.
∫ 20∫ 2y
0 (x+1)dxdy.
4. Evaluate the iterated integral and then write the iterated integral with the order ofintegration reversed.
∫ 30∫ y
0 sin(x) dxdy.
5. Evaluate the iterated integral and then write the iterated integral with the order ofintegration reversed.
∫ 10∫ y
0 exp(y) dxdy.