19.4. EXERCISES 359

The first listed cylinder intersects the xy plane in the disk, x2 + y2 ≤ 1. What is thevolume of the three dimensional region which is between this disk and the two surfaces,z =√

1− x2 and z =−√

1− x2? An iterated integral for the volume is

∫ 1

−1

∫ √1−x2

−√

1−x2

∫ √1−x2

−√

1−x2dzdydx =

163.

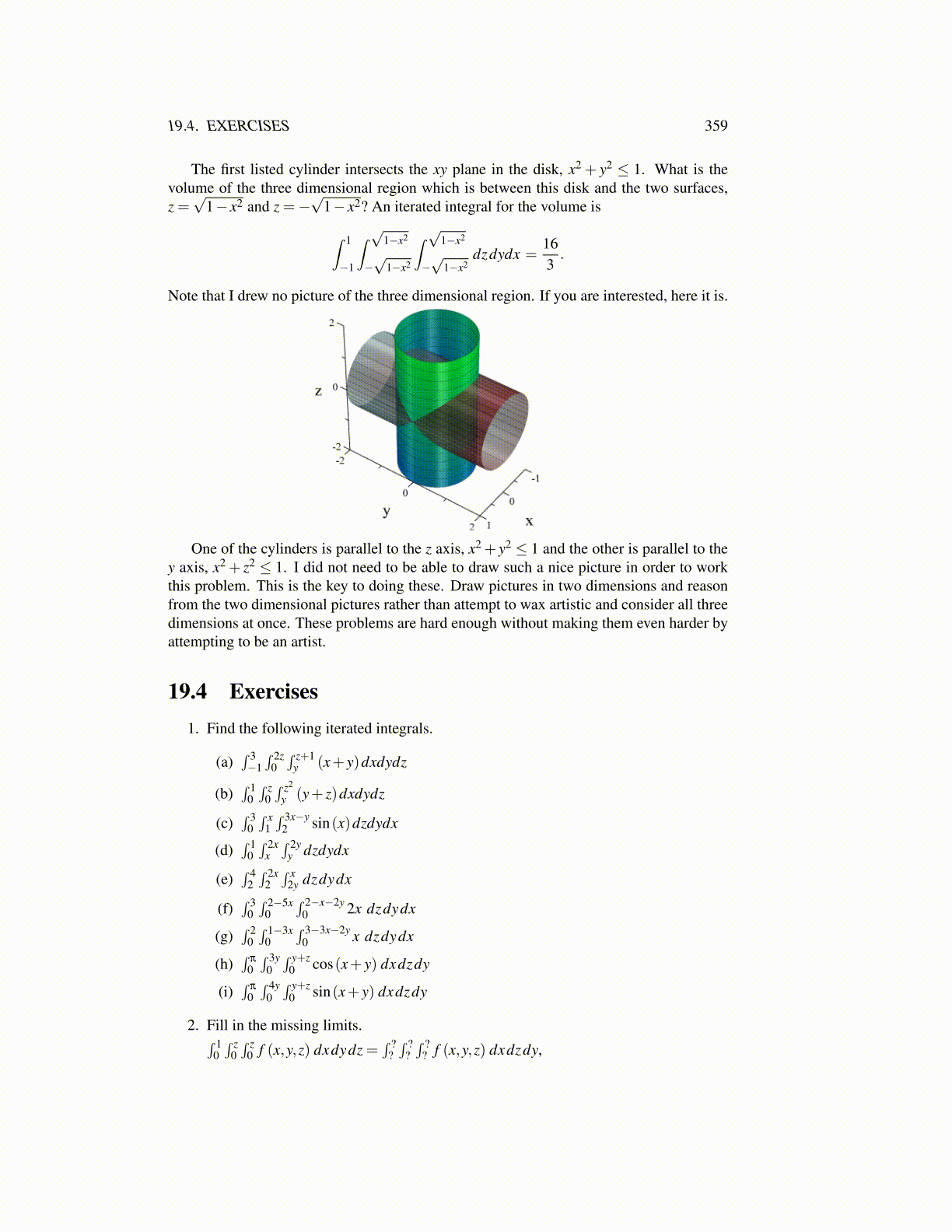
Note that I drew no picture of the three dimensional region. If you are interested, here it is.

One of the cylinders is parallel to the z axis, x2 + y2 ≤ 1 and the other is parallel to they axis, x2 + z2 ≤ 1. I did not need to be able to draw such a nice picture in order to workthis problem. This is the key to doing these. Draw pictures in two dimensions and reasonfrom the two dimensional pictures rather than attempt to wax artistic and consider all threedimensions at once. These problems are hard enough without making them even harder byattempting to be an artist.

19.4 Exercises1. Find the following iterated integrals.

(a)∫ 3−1∫ 2z

0∫ z+1

y (x+ y)dxdydz

(b)∫ 1

0∫ z

0∫ z2

y (y+ z)dxdydz

(c)∫ 3

0∫ x

1∫ 3x−y

2 sin(x)dzdydx

(d)∫ 1

0∫ 2x

x∫ 2y

y dzdydx

(e)∫ 4

2∫ 2x

2∫ x

2y dzdydx

(f)∫ 3

0∫ 2−5x

0∫ 2−x−2y

0 2x dzdydx

(g)∫ 2

0∫ 1−3x

0∫ 3−3x−2y

0 x dzdydx

(h)∫

π

0∫ 3y

0∫ y+z

0 cos(x+ y) dxdzdy

(i)∫

π

0∫ 4y

0∫ y+z

0 sin(x+ y) dxdzdy

2. Fill in the missing limits.∫ 10∫ z

0∫ z

0 f (x,y,z) dxdydz =∫ ?

?∫ ?

?∫ ?

? f (x,y,z) dxdzdy,