362 CHAPTER 19. THE RIEMANNN INTEGRAL ON Rn

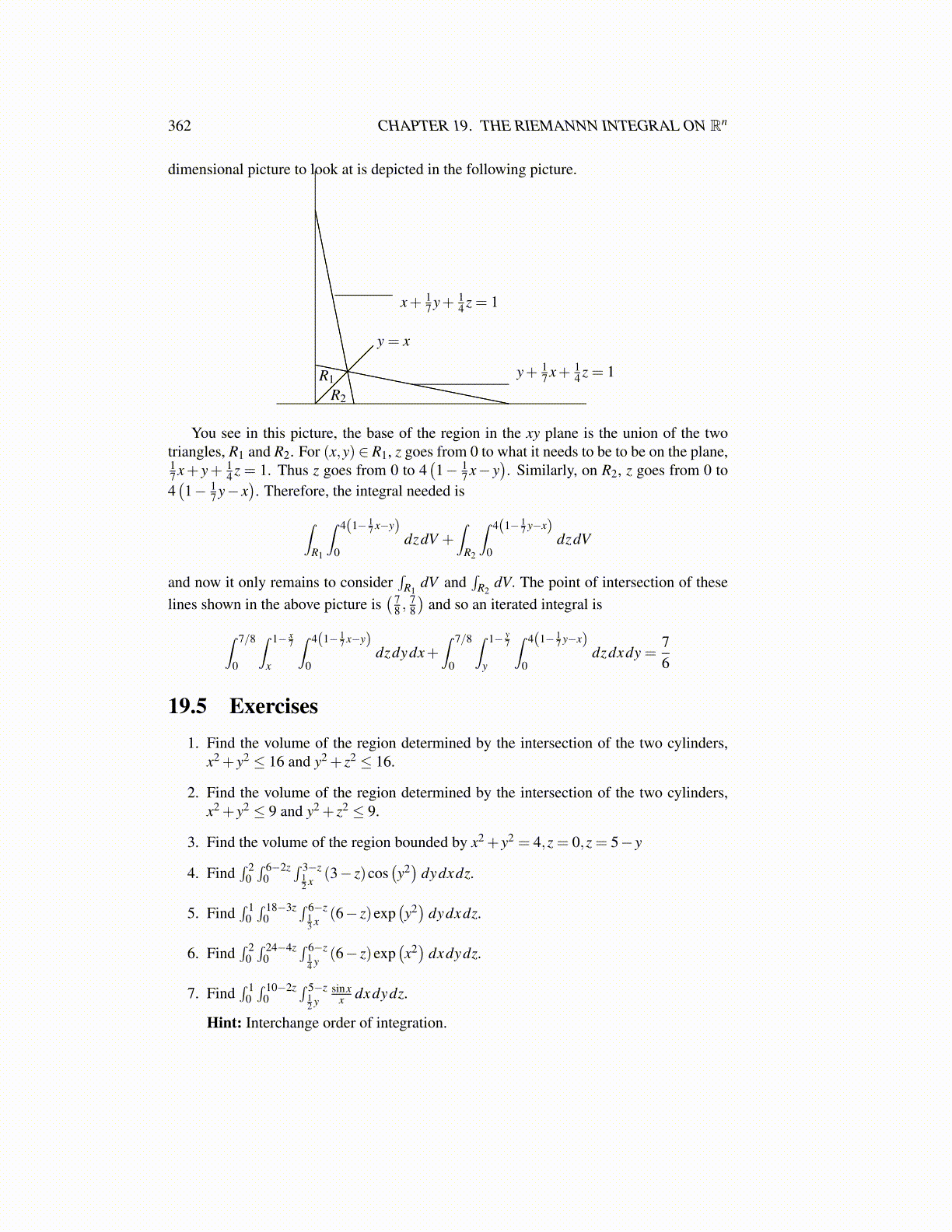
dimensional picture to look at is depicted in the following picture.

x+ 17 y+ 1

4 z = 1

y+ 17 x+ 1

4 z = 1R1R2

y = x

You see in this picture, the base of the region in the xy plane is the union of the twotriangles, R1 and R2. For (x,y)∈ R1, z goes from 0 to what it needs to be to be on the plane,17 x+ y+ 1

4 z = 1. Thus z goes from 0 to 4(1− 1

7 x− y). Similarly, on R2, z goes from 0 to

4(1− 1

7 y− x). Therefore, the integral needed is

∫R1

∫ 4(1− 17 x−y)

0dzdV +

∫R2

∫ 4(1− 17 y−x)

0dzdV

and now it only remains to consider∫

R1dV and

∫R2

dV. The point of intersection of theselines shown in the above picture is

( 78 ,

78

)and so an iterated integral is

∫ 7/8

0

∫ 1− x7

x

∫ 4(1− 17 x−y)

0dzdydx+

∫ 7/8

0

∫ 1− y7

y

∫ 4(1− 17 y−x)

0dzdxdy =

76

19.5 Exercises1. Find the volume of the region determined by the intersection of the two cylinders,

x2 + y2 ≤ 16 and y2 + z2 ≤ 16.

2. Find the volume of the region determined by the intersection of the two cylinders,x2 + y2 ≤ 9 and y2 + z2 ≤ 9.

3. Find the volume of the region bounded by x2 + y2 = 4,z = 0,z = 5− y

4. Find∫ 2

0∫ 6−2z

0∫ 3−z

12 x

(3− z)cos(y2)

dydxdz.

5. Find∫ 1

0∫ 18−3z

0∫ 6−z

13 x

(6− z)exp(y2)

dydxdz.

6. Find∫ 2

0∫ 24−4z

0∫ 6−z

14 y

(6− z)exp(x2)

dxdydz.

7. Find∫ 1

0∫ 10−2z

0∫ 5−z

12 y

sinxx dxdydz.

Hint: Interchange order of integration.