Chapter 20
The Integral In OtherCoordinates
20.1 Polar CoordinatesRecall the relation between the rectangular coordinates and polar coordinates is
x(r,θ)≡
(xy
)=
(r cos(θ)r sin(θ)
), r ≥ 0, θ ∈ [0,2π)
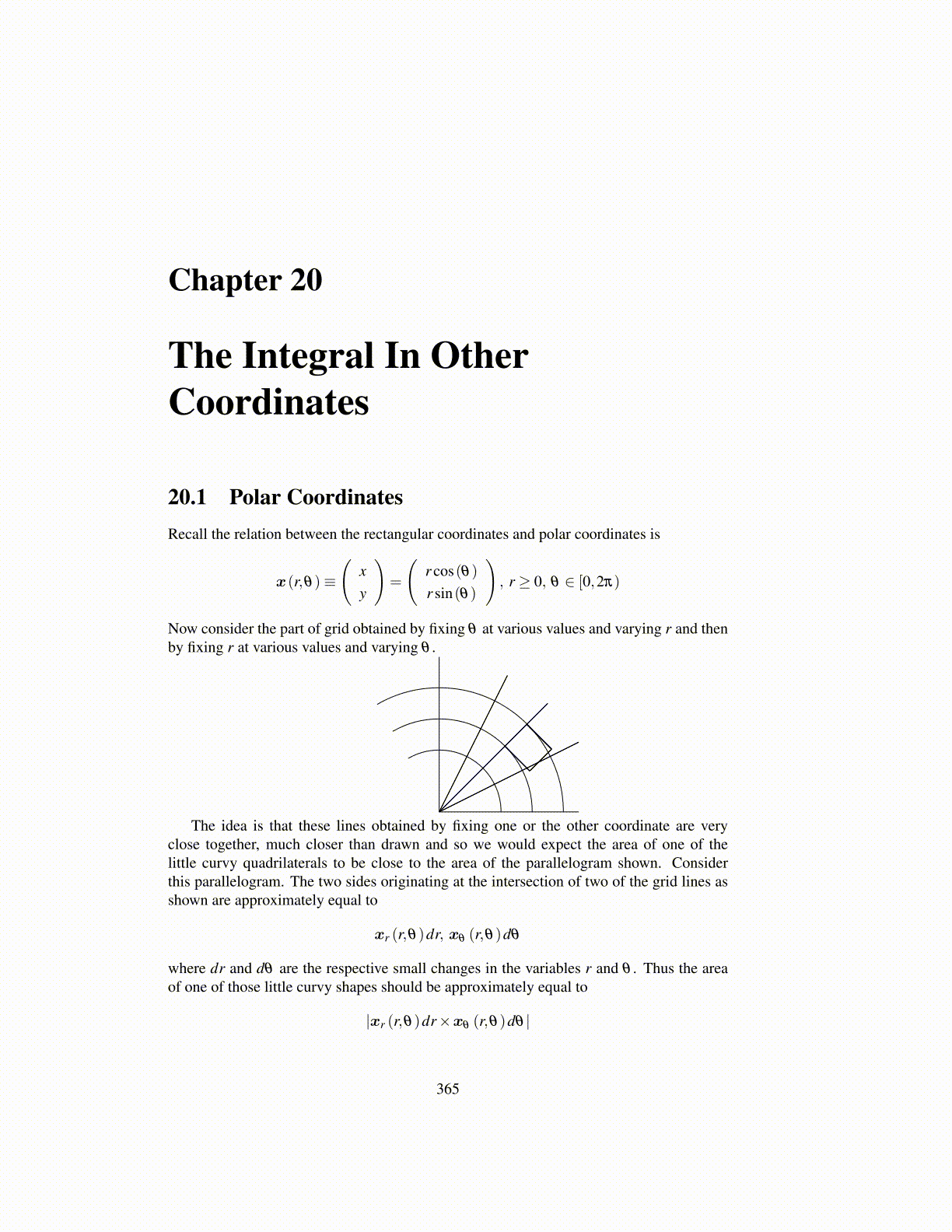
Now consider the part of grid obtained by fixing θ at various values and varying r and thenby fixing r at various values and varying θ .
The idea is that these lines obtained by fixing one or the other coordinate are veryclose together, much closer than drawn and so we would expect the area of one of thelittle curvy quadrilaterals to be close to the area of the parallelogram shown. Considerthis parallelogram. The two sides originating at the intersection of two of the grid lines asshown are approximately equal to
xr (r,θ)dr, xθ (r,θ)dθ
where dr and dθ are the respective small changes in the variables r and θ . Thus the areaof one of those little curvy shapes should be approximately equal to
|xr (r,θ)dr×xθ (r,θ)dθ |
365