390 CHAPTER 21. THE INTEGRAL ON TWO DIMENSIONAL SURFACES IN R3
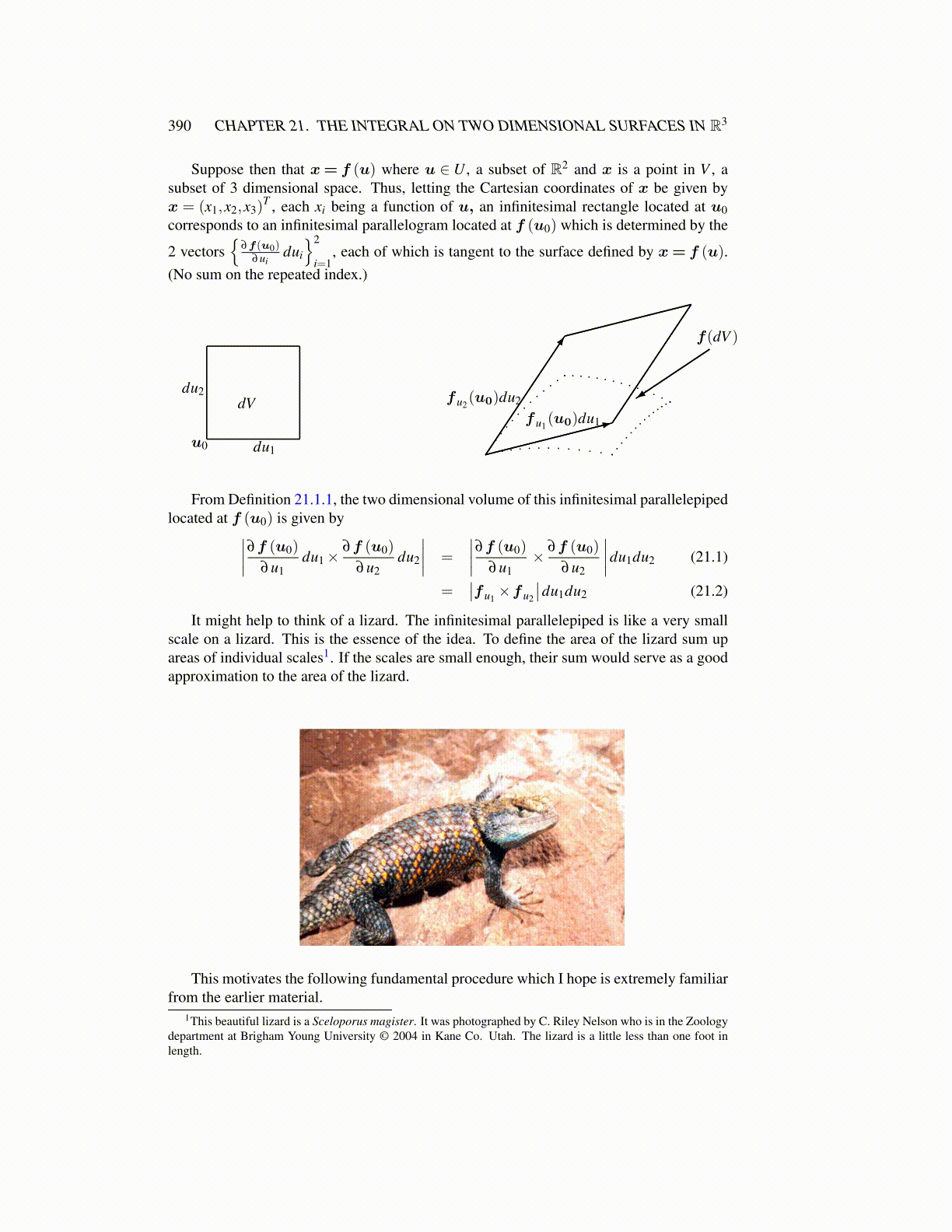
Suppose then that x= f (u) where u ∈ U , a subset of R2 and x is a point in V , asubset of 3 dimensional space. Thus, letting the Cartesian coordinates of x be given byx = (x1,x2,x3)
T , each xi being a function of u, an infinitesimal rectangle located at u0corresponds to an infinitesimal parallelogram located at f (u0) which is determined by the
2 vectors{
∂f(u0)∂ui
dui
}2
i=1, each of which is tangent to the surface defined by x= f (u).
(No sum on the repeated index.)
dV
u0
du2
du1
fu2(u0)du2
fu1(u0)du1
f(dV )
From Definition 21.1.1, the two dimensional volume of this infinitesimal parallelepipedlocated at f (u0) is given by∣∣∣∣∂f (u0)
∂u1du1×
∂f (u0)
∂u2du2
∣∣∣∣ =
∣∣∣∣∂f (u0)
∂u1× ∂f (u0)
∂u2
∣∣∣∣du1du2 (21.1)
=∣∣fu1×fu2
∣∣du1du2 (21.2)
It might help to think of a lizard. The infinitesimal parallelepiped is like a very smallscale on a lizard. This is the essence of the idea. To define the area of the lizard sum upareas of individual scales1. If the scales are small enough, their sum would serve as a goodapproximation to the area of the lizard.
This motivates the following fundamental procedure which I hope is extremely familiarfrom the earlier material.
1This beautiful lizard is a Sceloporus magister. It was photographed by C. Riley Nelson who is in the Zoologydepartment at Brigham Young University © 2004 in Kane Co. Utah. The lizard is a little less than one foot inlength.