104 CHAPTER 3. DETERMINANTS
3.5 Block Multiplication of Matrices
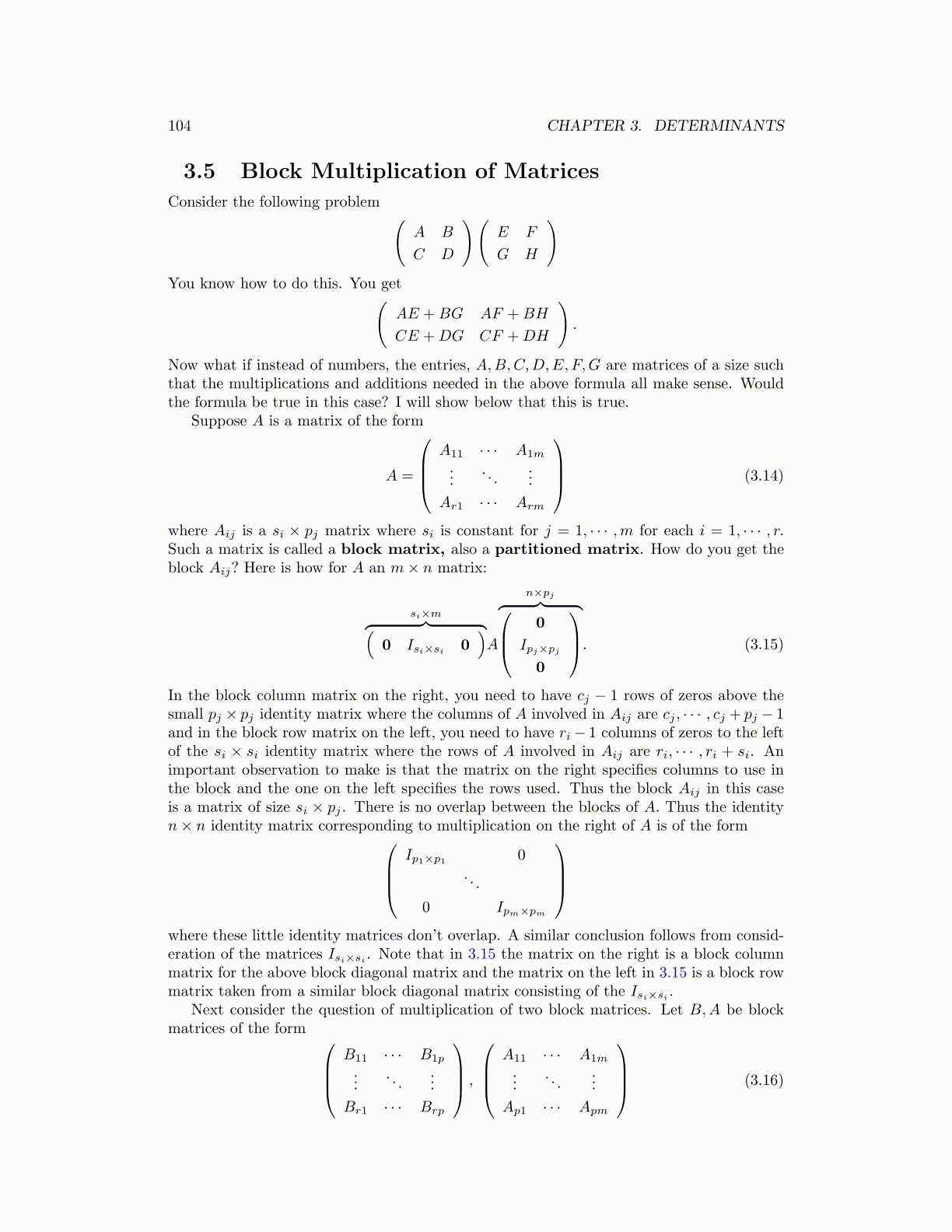
Consider the following problem (A B
C D
)(E F
G H
)You know how to do this. You get(
AE +BG AF +BH
CE +DG CF +DH
).
Now what if instead of numbers, the entries, A,B,C,D,E, F,G are matrices of a size suchthat the multiplications and additions needed in the above formula all make sense. Wouldthe formula be true in this case? I will show below that this is true.
Suppose A is a matrix of the form
A =
A11 · · · A1m
.... . .
...
Ar1 · · · Arm
(3.14)
where Aij is a si × pj matrix where si is constant for j = 1, · · · ,m for each i = 1, · · · , r.Such a matrix is called a block matrix, also a partitioned matrix. How do you get theblock Aij? Here is how for A an m× n matrix:
si×m︷ ︸︸ ︷(0 Isi×si 0
)A
n×pj︷ ︸︸ ︷ 0
Ipj×pj
0
. (3.15)
In the block column matrix on the right, you need to have cj − 1 rows of zeros above thesmall pj × pj identity matrix where the columns of A involved in Aij are cj , · · · , cj + pj − 1and in the block row matrix on the left, you need to have ri − 1 columns of zeros to the leftof the si × si identity matrix where the rows of A involved in Aij are ri, · · · , ri + si. Animportant observation to make is that the matrix on the right specifies columns to use inthe block and the one on the left specifies the rows used. Thus the block Aij in this caseis a matrix of size si × pj . There is no overlap between the blocks of A. Thus the identityn× n identity matrix corresponding to multiplication on the right of A is of the form
Ip1×p10
. . .
0 Ipm×pm
where these little identity matrices don’t overlap. A similar conclusion follows from consid-eration of the matrices Isi×si . Note that in 3.15 the matrix on the right is a block columnmatrix for the above block diagonal matrix and the matrix on the left in 3.15 is a block rowmatrix taken from a similar block diagonal matrix consisting of the Isi×si .
Next consider the question of multiplication of two block matrices. Let B,A be blockmatrices of the form
B11 · · · B1p
.... . .
...
Br1 · · · Brp
,
A11 · · · A1m
.... . .
...
Ap1 · · · Apm
(3.16)