6.5. TRACE AND DETERMINANT 167
are the corresponding eigenvectors. Permuting these columns, one can cause the eigenvaluesto appear in any order on the diagonal. ■
The converse for the above theorems about normal and Hermitian matrices is also true.That is, the Hermitian matrices, (A = A∗) are exactly those for which there is a unitary Usuch that U∗AU is a real diagonal matrix. The normal matrices are exactly those for whichthere is a unitary U such that U∗AU is a diagonal matrix, maybe not real.
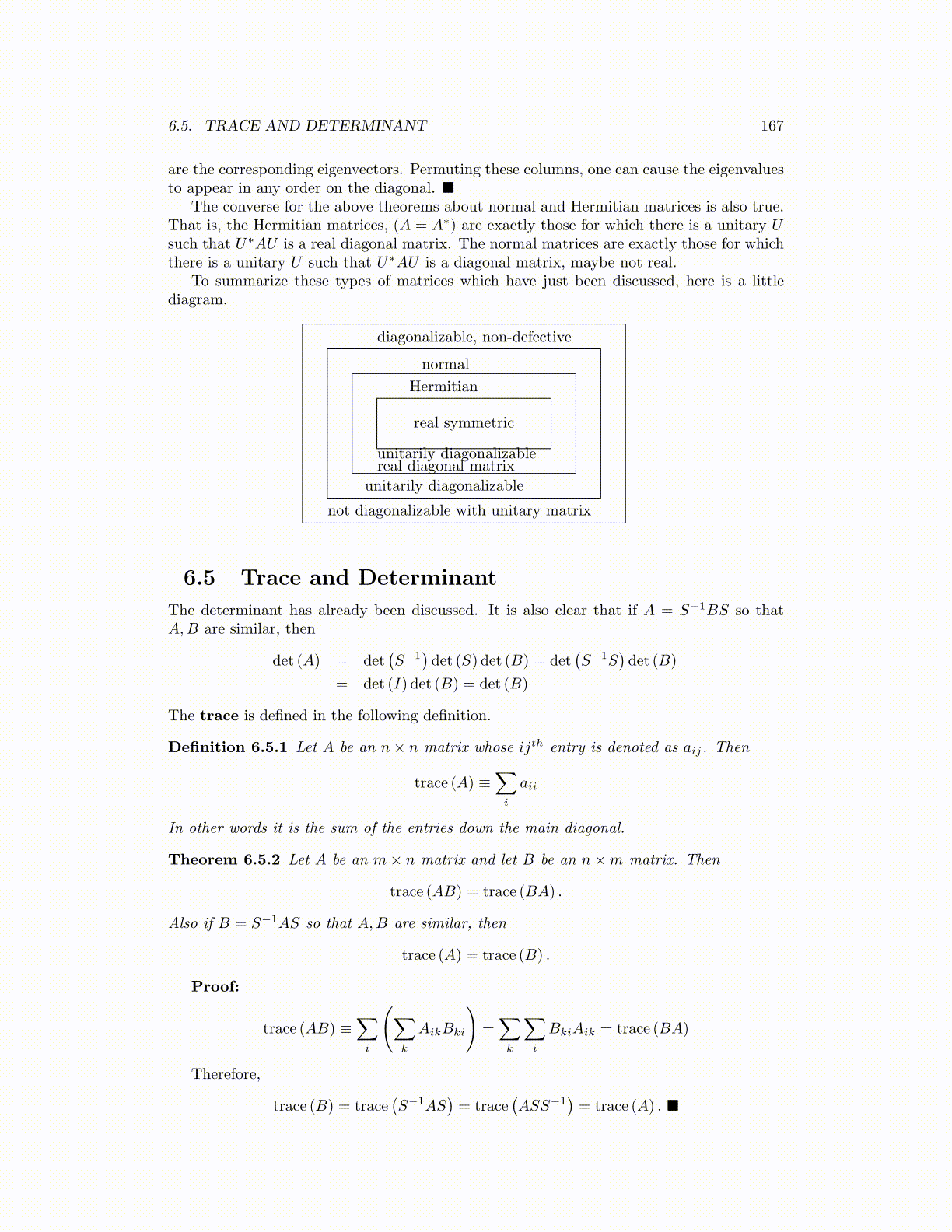
To summarize these types of matrices which have just been discussed, here is a littlediagram.
real symmetric
Hermitian
unitarily diagonalizablereal diagonal matrix
normal
unitarily diagonalizable
diagonalizable, non-defective
not diagonalizable with unitary matrix
6.5 Trace and Determinant
The determinant has already been discussed. It is also clear that if A = S−1BS so thatA,B are similar, then
det (A) = det(S−1
)det (S) det (B) = det
(S−1S
)det (B)
= det (I) det (B) = det (B)
The trace is defined in the following definition.
Definition 6.5.1 Let A be an n× n matrix whose ijth entry is denoted as aij. Then
trace (A) ≡∑i
aii
In other words it is the sum of the entries down the main diagonal.
Theorem 6.5.2 Let A be an m× n matrix and let B be an n×m matrix. Then
trace (AB) = trace (BA) .
Also if B = S−1AS so that A,B are similar, then
trace (A) = trace (B) .
Proof:
trace (AB) ≡∑i
(∑k
AikBki
)=∑k
∑i
BkiAik = trace (BA)
Therefore,
trace (B) = trace(S−1AS
)= trace
(ASS−1
)= trace (A) . ■