1.6. THE FUNDAMENTAL THEOREM OF ALGEBRA 17

•0

Ar r large• a0Ar

r small

Thus it is reasonable to believe that for some r dur-ing this shrinking process, the set Ar must hit 0. Itfollows that p (z) = 0 for some z.

For example, consider the polynomial x3+x+1+i.It has no real zeros. However, you could let z = r (cos t+ i sin t) and insert this into thepolynomial. Thus you would want to find a point where

(r (cos t+ i sin t))3+ r (cos t+ i sin t) + 1 + i = 0 + 0i

Expanding this expression on the left to write it in terms of real and imaginary parts, youget on the left

r3 cos3 t− 3r3 cos t sin2 t+ r cos t+ 1 + i(3r3 cos2 t sin t− r3 sin3 t+ r sin t+ 1

)Thus you need to have both the real and imaginary parts equal to 0. In other words, youneed to have (0, 0) =(

r3 cos3 t− 3r3 cos t sin2 t+ r cos t+ 1, 3r3 cos2 t sin t− r3 sin3 t+ r sin t+ 1)

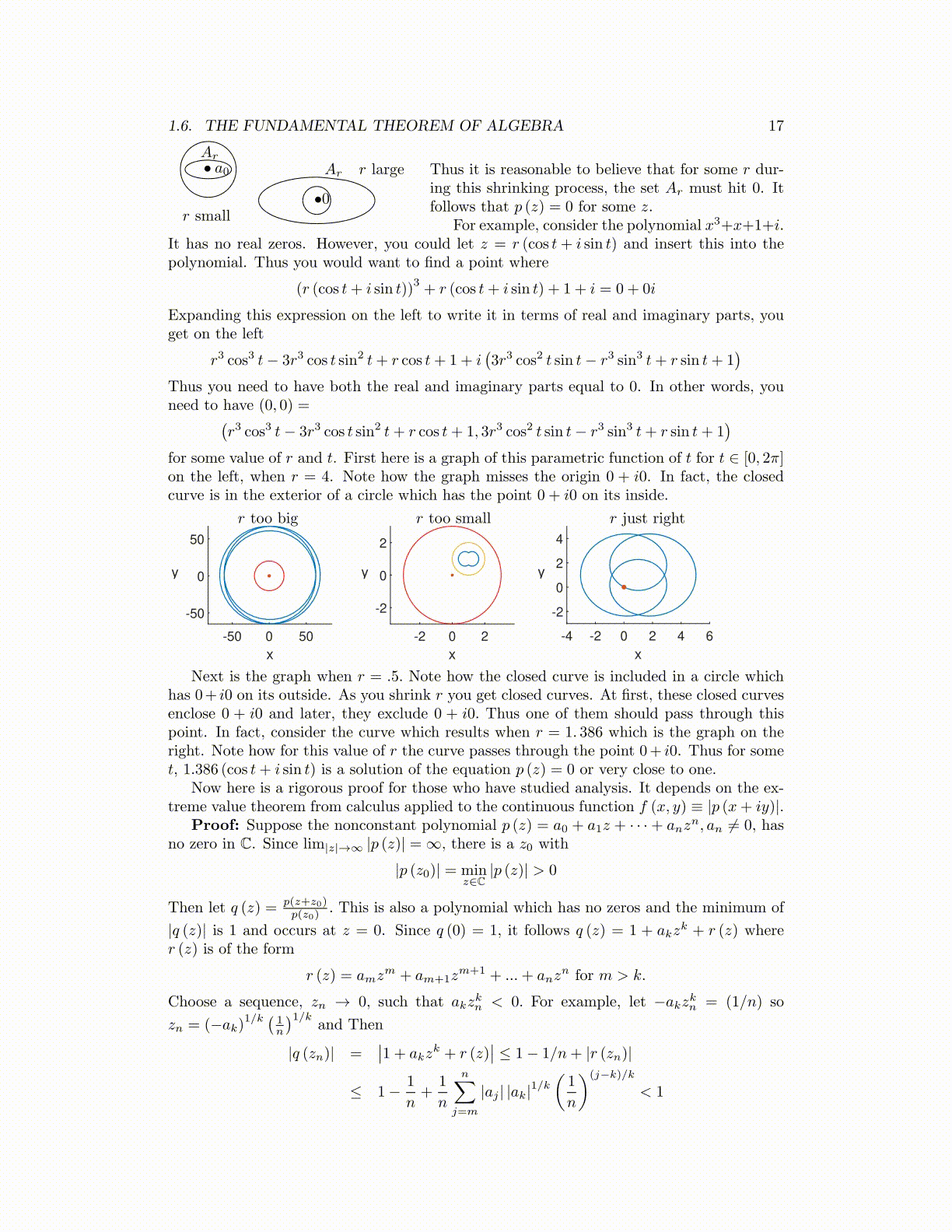
for some value of r and t. First here is a graph of this parametric function of t for t ∈ [0, 2π]on the left, when r = 4. Note how the graph misses the origin 0 + i0. In fact, the closedcurve is in the exterior of a circle which has the point 0 + i0 on its inside.

-50 0 50

x

-50

0

50

y

-2 0 2

x

-2

0

2

y

-4 -2 0 2 4 6

x

-2

0

2

4

y

r too big r too small r just right

Next is the graph when r = .5. Note how the closed curve is included in a circle whichhas 0+ i0 on its outside. As you shrink r you get closed curves. At first, these closed curvesenclose 0 + i0 and later, they exclude 0 + i0. Thus one of them should pass through thispoint. In fact, consider the curve which results when r = 1. 386 which is the graph on theright. Note how for this value of r the curve passes through the point 0+ i0. Thus for somet, 1.386 (cos t+ i sin t) is a solution of the equation p (z) = 0 or very close to one.

Now here is a rigorous proof for those who have studied analysis. It depends on the ex-treme value theorem from calculus applied to the continuous function f (x, y) ≡ |p (x+ iy)|.

Proof: Suppose the nonconstant polynomial p (z) = a0 + a1z + · · ·+ anzn, an ̸= 0, has

no zero in C. Since lim|z|→∞ |p (z)| = ∞, there is a z0 with

|p (z0)| = minz∈C

|p (z)| > 0

Then let q (z) = p(z+z0)p(z0)

. This is also a polynomial which has no zeros and the minimum of

|q (z)| is 1 and occurs at z = 0. Since q (0) = 1, it follows q (z) = 1 + akzk + r (z) where

r (z) is of the form

r (z) = amzm + am+1z

m+1 + ...+ anzn for m > k.

Choose a sequence, zn → 0, such that akzkn < 0. For example, let −akzkn = (1/n) so

zn = (−ak)1/k(1n

)1/kand Then

|q (zn)| =∣∣1 + akz

k + r (z)∣∣ ≤ 1− 1/n+ |r (zn)|

≤ 1− 1

n+

1

n

n∑j=m

|aj | |ak|1/k(1

n

)(j−k)/k

< 1