12.1. OUTER MEASURES 271

S⋂

AC⋂BC

S⋂

AC⋂B

S⋂

A⋂

B

S⋂

A⋂

BC

A

B

S

Since µ is subadditive,

µ (S)≤ µ(S∩A∩BC)+µ (A∩B∩S)+µ

(S∩B∩AC)+µ

(S∩AC ∩BC) .

Now using A,B ∈S ,

µ (S) ≤ µ(S∩A∩BC)+µ (S∩A∩B)+µ

(S∩AC ∩B

)+µ

(S∩AC ∩BC)

= µ (S∩A)+µ(S∩AC)= µ (S)

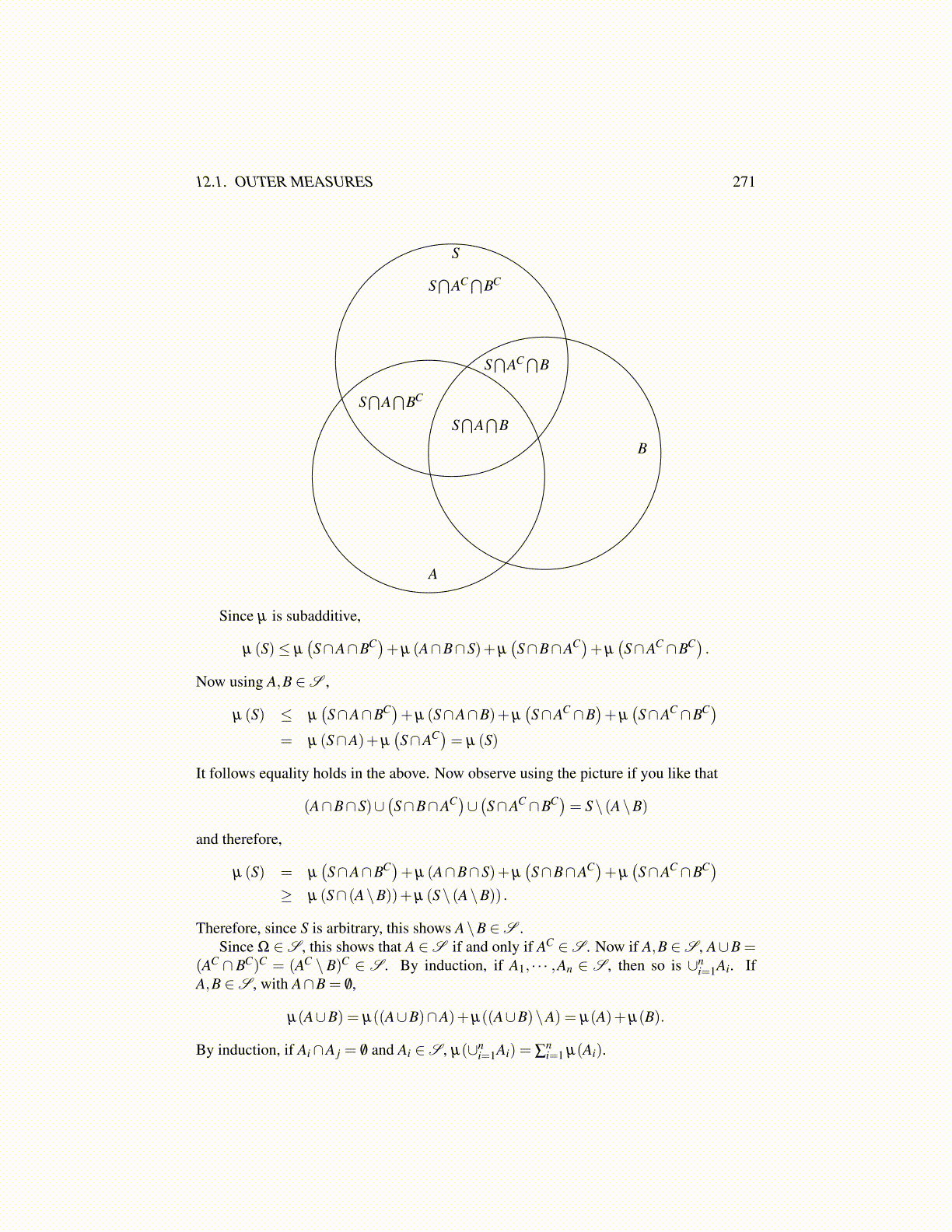
It follows equality holds in the above. Now observe using the picture if you like that

(A∩B∩S)∪(S∩B∩AC)∪ (S∩AC ∩BC)= S\ (A\B)

and therefore,

µ (S) = µ(S∩A∩BC)+µ (A∩B∩S)+µ

(S∩B∩AC)+µ

(S∩AC ∩BC)

≥ µ (S∩ (A\B))+µ (S\ (A\B)) .

Therefore, since S is arbitrary, this shows A\B ∈S .Since Ω ∈S , this shows that A ∈S if and only if AC ∈S . Now if A,B ∈S , A∪B =

(AC ∩ BC)C = (AC \ B)C ∈ S . By induction, if A1, · · · ,An ∈ S , then so is ∪ni=1Ai. If

A,B ∈S , with A∩B = /0,

µ(A∪B) = µ((A∪B)∩A)+µ((A∪B)\A) = µ(A)+µ(B).

By induction, if Ai∩A j = /0 and Ai ∈S , µ(∪ni=1Ai) = ∑

ni=1 µ(Ai).