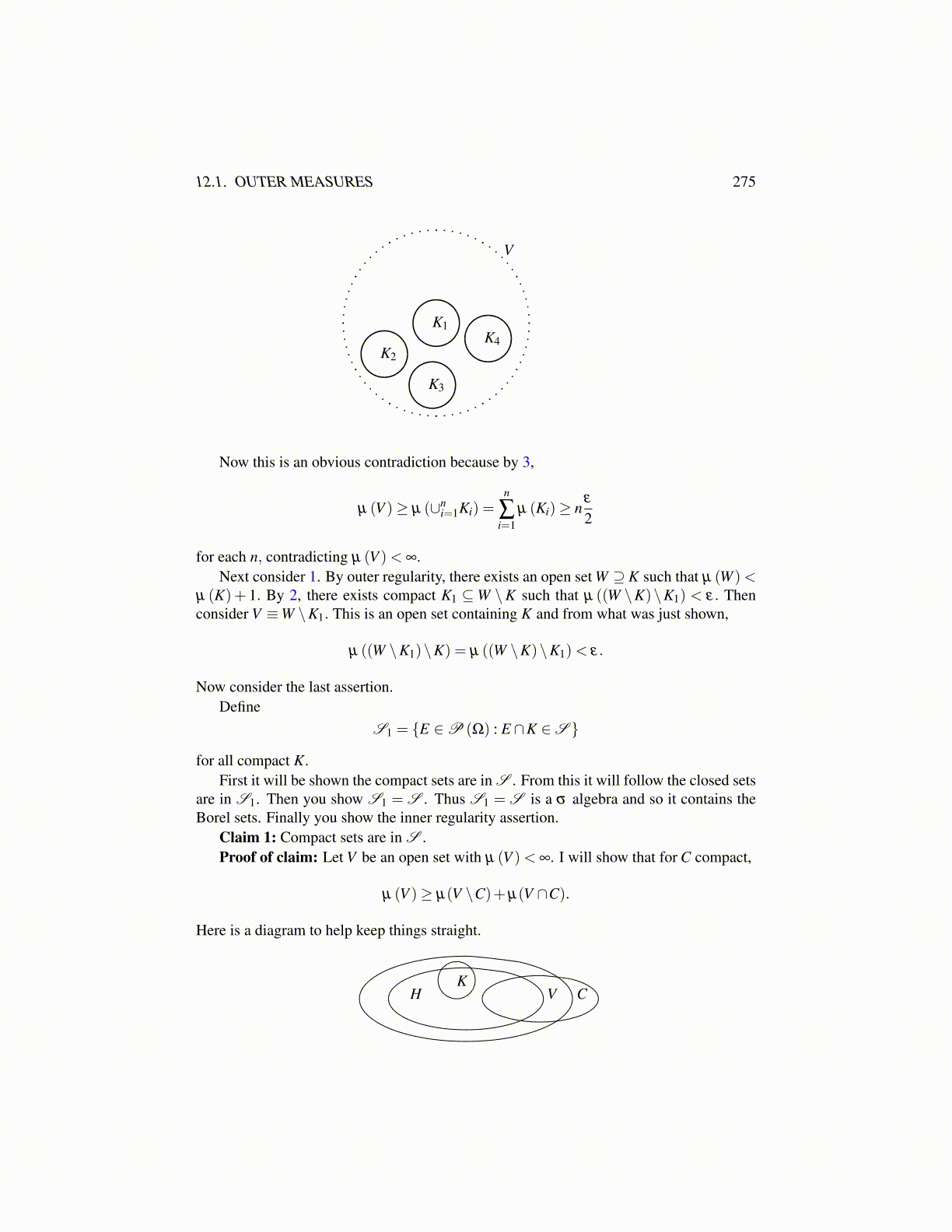
12.1. OUTER MEASURES 275
V
K1K4
K2
K3
Now this is an obvious contradiction because by 3,
µ (V )≥ µ (∪ni=1Ki) =
n
∑i=1
µ (Ki)≥ nε
2
for each n, contradicting µ (V )< ∞.Next consider 1. By outer regularity, there exists an open set W ⊇ K such that µ (W )<
µ (K)+ 1. By 2, there exists compact K1 ⊆W \K such that µ ((W \K)\K1) < ε. Thenconsider V ≡W \K1. This is an open set containing K and from what was just shown,
µ ((W \K1)\K) = µ ((W \K)\K1)< ε.
Now consider the last assertion.Define
S1 = {E ∈P (Ω) : E ∩K ∈S }
for all compact K.First it will be shown the compact sets are in S . From this it will follow the closed sets
are in S1. Then you show S1 = S . Thus S1 = S is a σ algebra and so it contains theBorel sets. Finally you show the inner regularity assertion.
Claim 1: Compact sets are in S .Proof of claim: Let V be an open set with µ (V )< ∞. I will show that for C compact,
µ (V )≥ µ(V \C)+µ(V ∩C).
Here is a diagram to help keep things straight.
VH CK