4.3. FUNCTIONS OF RIEMANN INTEGRABLE FUNCTIONS 49
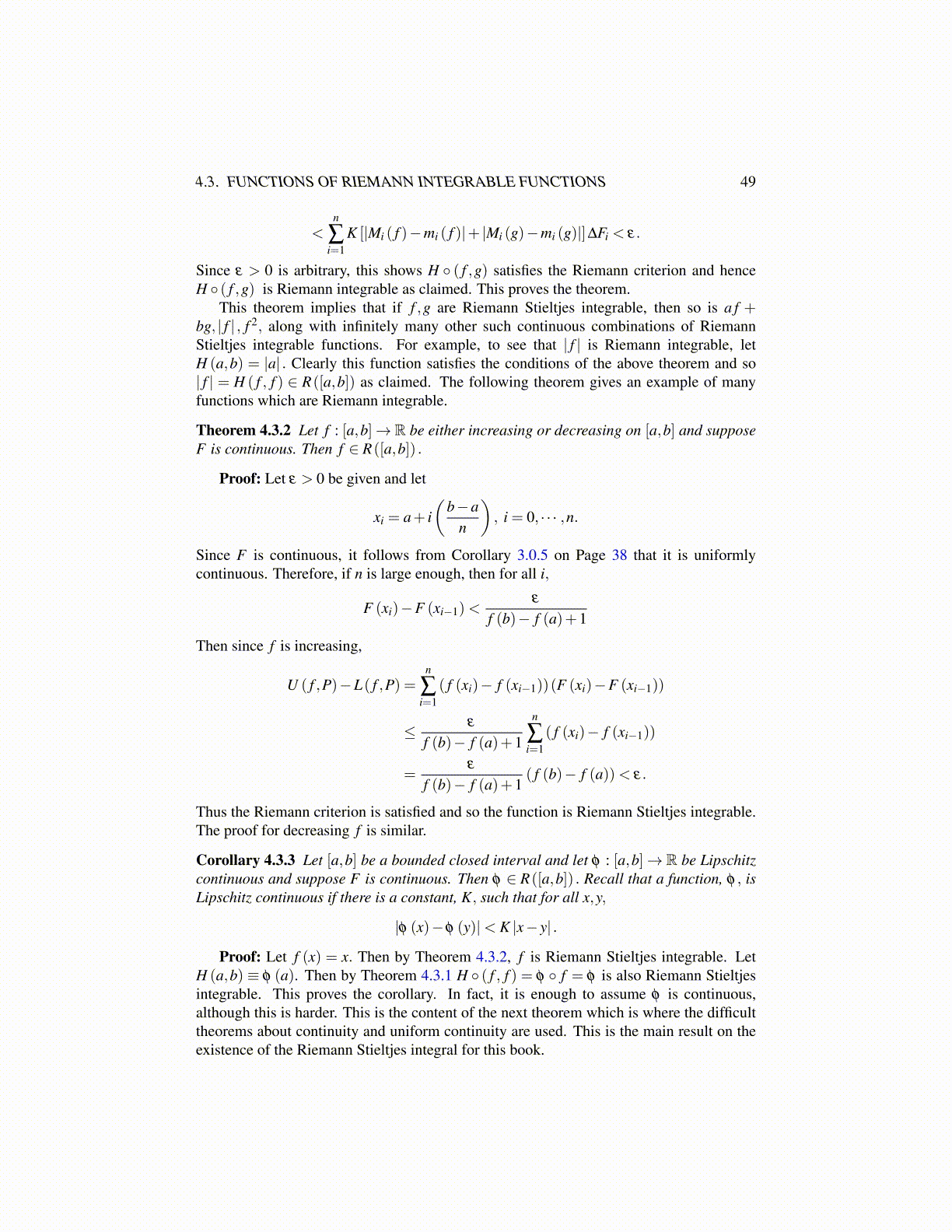
<n
∑i=1
K [|Mi ( f )−mi ( f )|+ |Mi (g)−mi (g)|]∆Fi < ε.
Since ε > 0 is arbitrary, this shows H ◦ ( f ,g) satisfies the Riemann criterion and henceH ◦ ( f ,g) is Riemann integrable as claimed. This proves the theorem.
This theorem implies that if f ,g are Riemann Stieltjes integrable, then so is a f +bg, | f | , f 2, along with infinitely many other such continuous combinations of RiemannStieltjes integrable functions. For example, to see that | f | is Riemann integrable, letH (a,b) = |a| . Clearly this function satisfies the conditions of the above theorem and so| f | = H ( f , f ) ∈ R([a,b]) as claimed. The following theorem gives an example of manyfunctions which are Riemann integrable.
Theorem 4.3.2 Let f : [a,b]→ R be either increasing or decreasing on [a,b] and supposeF is continuous. Then f ∈ R([a,b]) .
Proof: Let ε > 0 be given and let
xi = a+ i(
b−an
), i = 0, · · · ,n.
Since F is continuous, it follows from Corollary 3.0.5 on Page 38 that it is uniformlycontinuous. Therefore, if n is large enough, then for all i,
F (xi)−F (xi−1)<ε
f (b)− f (a)+1
Then since f is increasing,
U ( f ,P)−L( f ,P) =n
∑i=1
( f (xi)− f (xi−1))(F (xi)−F (xi−1))
≤ ε
f (b)− f (a)+1
n
∑i=1
( f (xi)− f (xi−1))
=ε
f (b)− f (a)+1( f (b)− f (a))< ε.
Thus the Riemann criterion is satisfied and so the function is Riemann Stieltjes integrable.The proof for decreasing f is similar.
Corollary 4.3.3 Let [a,b] be a bounded closed interval and let φ : [a,b]→ R be Lipschitzcontinuous and suppose F is continuous. Then φ ∈ R([a,b]) . Recall that a function, φ , isLipschitz continuous if there is a constant, K, such that for all x,y,
|φ (x)−φ (y)|< K |x− y| .
Proof: Let f (x) = x. Then by Theorem 4.3.2, f is Riemann Stieltjes integrable. LetH (a,b) ≡ φ (a). Then by Theorem 4.3.1 H ◦ ( f , f ) = φ ◦ f = φ is also Riemann Stieltjesintegrable. This proves the corollary. In fact, it is enough to assume φ is continuous,although this is harder. This is the content of the next theorem which is where the difficulttheorems about continuity and uniform continuity are used. This is the main result on theexistence of the Riemann Stieltjes integral for this book.