6.3. CONTINUOUS FUNCTIONS 99
Note that if r = 0 then B(x,r) = /0, the empty set. This is because if y ∈ Fn, |x−y| ≥ 0 andso y /∈ B(x,0) . Since /0 has no points in it, it must be open because every point in it, (Thereare none.) satisfies the desired property of being an interior point.
Now suppose y /∈ D(x,r) . Then |x−y|> r and defining δ ≡ |x−y|− r, it follows thatif z ∈ B(y,δ ) , then by the triangle inequality,
|x− z| ≥ |x−y|− |y− z|> |x−y|−δ
= |x−y|− (|x−y|− r) = r
and this shows that B(y,δ )⊆ Fn \D(x,r) . Since y was an arbitrary point in Fn \D(x,r) , itfollows Fn \D(x,r) is an open set which shows from the definition that D(x,r) is a closedset as claimed.
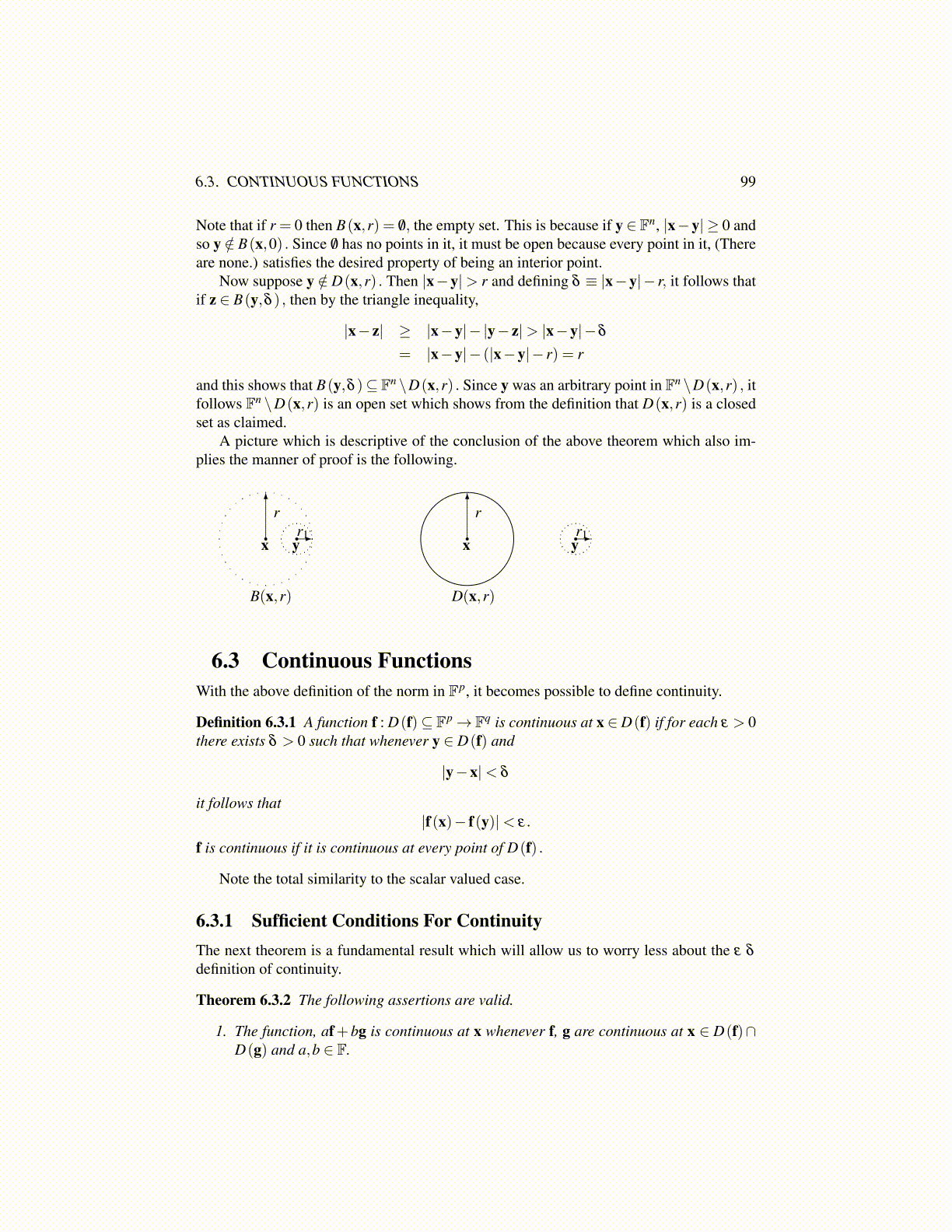
A picture which is descriptive of the conclusion of the above theorem which also im-plies the manner of proof is the following.
yx
rr1
B(x,r)
yx
rr1
D(x,r)
6.3 Continuous FunctionsWith the above definition of the norm in Fp, it becomes possible to define continuity.
Definition 6.3.1 A function f : D(f)⊆ Fp→ Fq is continuous at x ∈D(f) if for each ε > 0there exists δ > 0 such that whenever y ∈ D(f) and
|y−x|< δ
it follows that|f(x)− f(y)|< ε.
f is continuous if it is continuous at every point of D(f) .
Note the total similarity to the scalar valued case.
6.3.1 Sufficient Conditions For ContinuityThe next theorem is a fundamental result which will allow us to worry less about the ε δ
definition of continuity.
Theorem 6.3.2 The following assertions are valid.
1. The function, af+bg is continuous at x whenever f, g are continuous at x ∈ D(f)∩D(g) and a,b ∈ F.