114 CHAPTER 4. LINEAR SPACES

If B1,B2 ∈ G then B1∩B2 = /0, (4.24)

G is maximal with respect to 4.23 and 4.24. (4.25)

By this is meant that if H is a collection of balls satisfying 4.23 and 4.24, then H cannotproperly contain G .

Proof: Let S denote a subset of F such that 4.23 and 4.24 are satisfied. Since k < M,4.23 is satisfied for some ball of S. Thus S ̸= /0. Partially order S with respect to setinclusion. Thus A ≺B for A ,B in S means that A ⊆B. By the Hausdorff maximaltheorem, there is a maximal chain in S denoted by C . Then let G be ∪C . If B1,B2 are inC , then since C is a chain, both B1,B2 are in some element of C and so B1∩B2 = /0. Themaximality of C is violated if there is any other element of S which properly contains G .■

Proposition 4.5.3 Let F be a collection of balls, and let

A≡ ∪{B : B ∈F} .

Suppose∞ > M ≡ sup{r : B(p,r) ∈F}> 0.

Then there exists G ⊆F such that G consists of balls whose closures are disjoint and

A⊆ ∪{B̂ : B ∈ G }

where for B = B(x,r) a ball, B̂ denotes the open ball B(x,5r).

Proof: Let G1 satisfy 4.23 - 4.25 for k = 2M3 .

Suppose G1, · · · ,Gm−1 have been chosen for m ≥ 2. Let Gi denote the collection ofclosures of the balls of Gi. Then let Fm be those balls of F , such that if B is one of theseballs, B has empty intersection with every closed ball of Gi for each i≤ m−1. Then usingLemma 4.5.2, let Gm be a maximal collection of balls from Fm with the property that eachball has radius larger than

( 23

)mM and their closures are disjoint. Let G ≡ ∪∞

k=1Gk. Thusthe closures of balls in G are disjoint. Let x ∈ B(p,r) ∈F \G . Choose m such that(

23

)m

M < r ≤(

23

)m−1

M

Then B(p,r) must have nonempty intersection with the closure of some ball from G1 ∪·· ·∪Gm because if it didn’t, then Gm would fail to be maximal. Denote by B(p0,r0) a ballin G1∪·· ·∪Gm whose closure has nonempty intersection with B(p,r). Thus both

r0,r >(

23

)m

M, so r ≤(

23

)m−1

M <32

r0

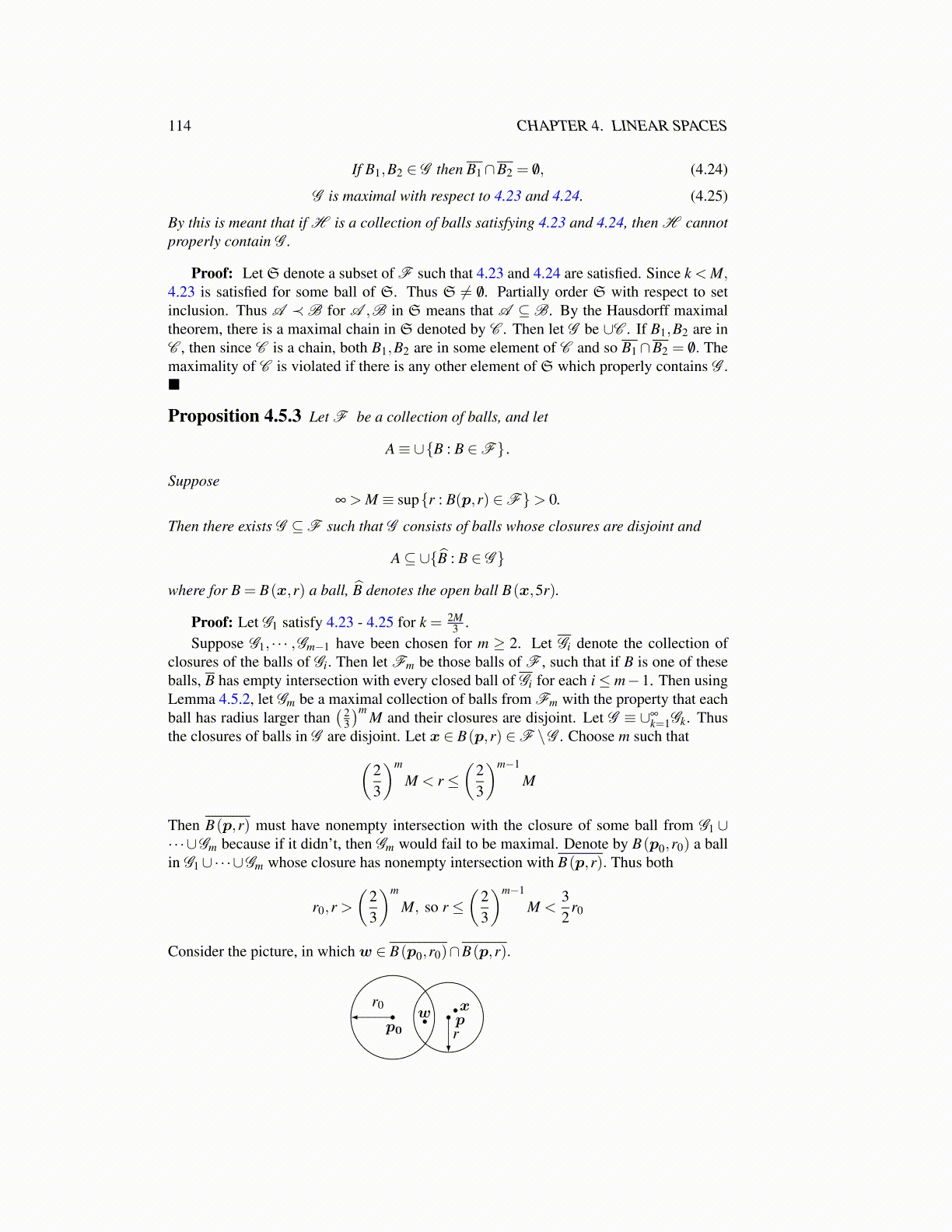
Consider the picture, in which w ∈ B(p0,r0)∩B(p,r).

wr0

p0 rpx