4.5. COVERING THEOREMS 115
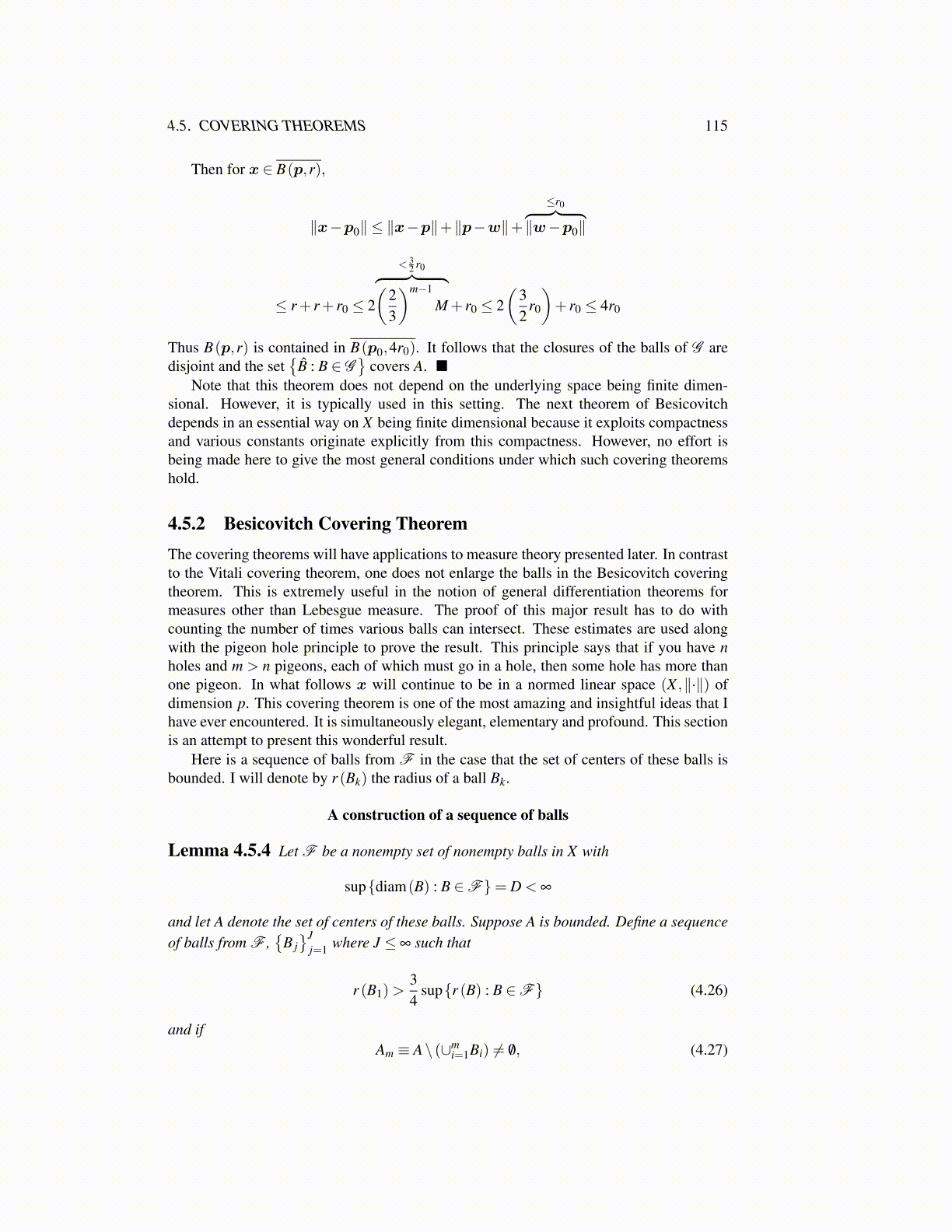
Then for x ∈ B(p,r),
∥x−p0∥ ≤ ∥x−p∥+∥p−w∥+
≤r0︷ ︸︸ ︷∥w−p0∥
≤ r+ r+ r0 ≤ 2
< 32 r0︷ ︸︸ ︷(
23
)m−1
M+ r0 ≤ 2(
32
r0
)+ r0 ≤ 4r0
Thus B(p,r) is contained in B(p0,4r0). It follows that the closures of the balls of G aredisjoint and the set
{B̂ : B ∈ G
}covers A. ■
Note that this theorem does not depend on the underlying space being finite dimen-sional. However, it is typically used in this setting. The next theorem of Besicovitchdepends in an essential way on X being finite dimensional because it exploits compactnessand various constants originate explicitly from this compactness. However, no effort isbeing made here to give the most general conditions under which such covering theoremshold.
4.5.2 Besicovitch Covering TheoremThe covering theorems will have applications to measure theory presented later. In contrastto the Vitali covering theorem, one does not enlarge the balls in the Besicovitch coveringtheorem. This is extremely useful in the notion of general differentiation theorems formeasures other than Lebesgue measure. The proof of this major result has to do withcounting the number of times various balls can intersect. These estimates are used alongwith the pigeon hole principle to prove the result. This principle says that if you have nholes and m > n pigeons, each of which must go in a hole, then some hole has more thanone pigeon. In what follows x will continue to be in a normed linear space (X ,∥·∥) ofdimension p. This covering theorem is one of the most amazing and insightful ideas that Ihave ever encountered. It is simultaneously elegant, elementary and profound. This sectionis an attempt to present this wonderful result.
Here is a sequence of balls from F in the case that the set of centers of these balls isbounded. I will denote by r (Bk) the radius of a ball Bk.
A construction of a sequence of balls
Lemma 4.5.4 Let F be a nonempty set of nonempty balls in X with
sup{diam(B) : B ∈F}= D < ∞
and let A denote the set of centers of these balls. Suppose A is bounded. Define a sequenceof balls from F ,
{B j}J
j=1 where J ≤ ∞ such that
r (B1)>34
sup{r (B) : B ∈F} (4.26)
and ifAm ≡ A\ (∪m
i=1Bi) ̸= /0, (4.27)