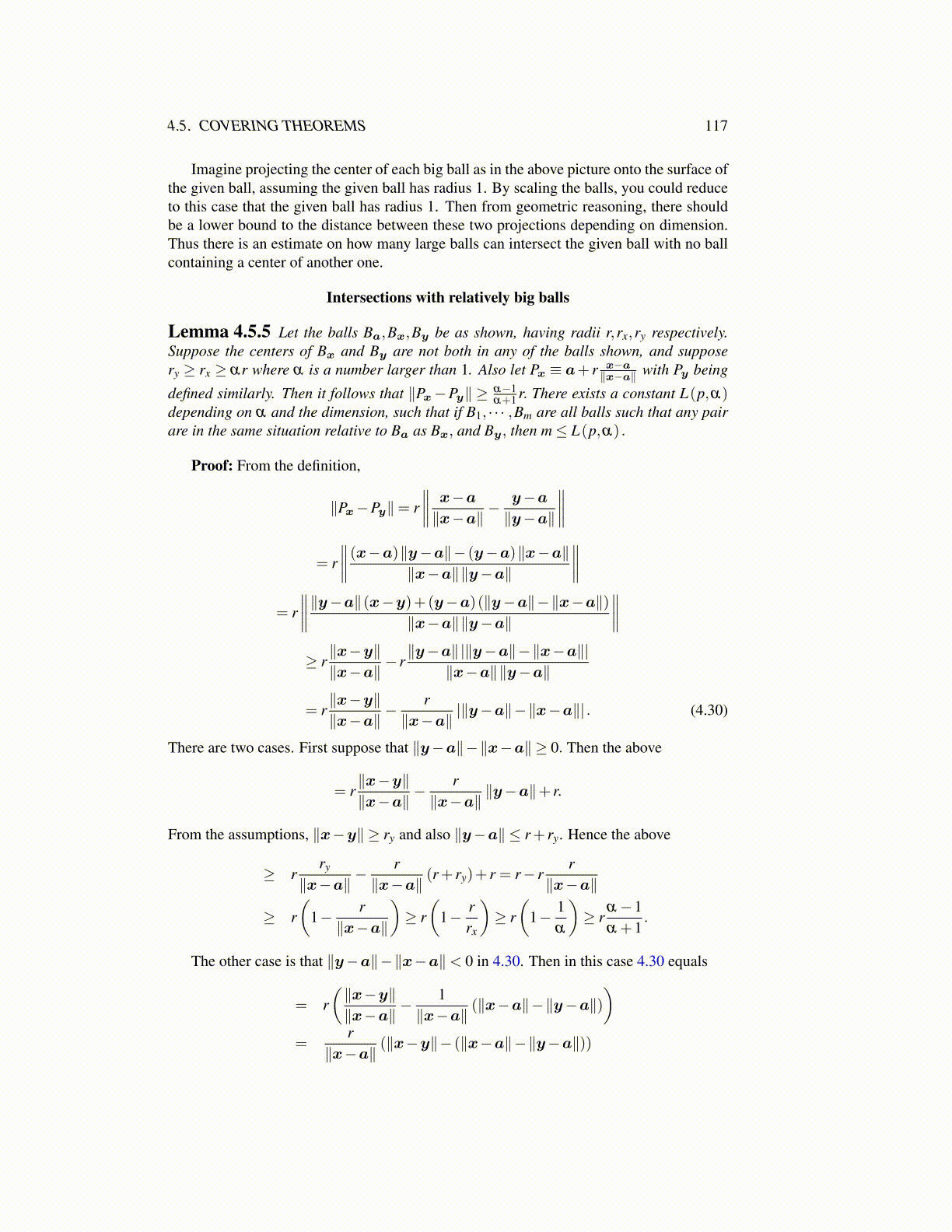
4.5. COVERING THEOREMS 117Imagine projecting the center of each big ball as in the above picture onto the surface ofthe given ball, assuming the given ball has radius 1. By scaling the balls, you could reduceto this case that the given ball has radius 1. Then from geometric reasoning, there shouldbe a lower bound to the distance between these two projections depending on dimension.Thus there is an estimate on how many large balls can intersect the given ball with no ballcontaining a center of another one.Intersections with relatively big ballsLemma 4.5.5 Let the balls Ba,Bz,By be as shown, having radii r,ry,ry respectively.Suppose the centers of By, and By are not both in any of the balls shown, and supposez—-ary > ry, = Or where & is a number larger than 1. Also let Py =a+ "Tal with Py beingdefined similarly. Then it follows that ||P, — Py|| > aor. There exists a constant L(p, 0)depending on a and the dimension, such that if B,,--- ,Bym are all balls such that any pairare in the same situation relative to Ba as By, and By, thenm < L(p,a).Proof: From the definition,xr-a y-a||P — Py|| =r"|||z—al| |ly—al(x —a)|ly—al|—(y—a) lla — a\|z—al||ly—a|-,|jz — all |ly—a|le=yll_lly—alliily all lle — allIla — all \|a — al ||y— a!xr-y r= feel thy a —le—alll (4.30)||z—al| ||a—alThere are two cases. First suppose that ||y—a'| — ||a — al] > 0. Then the abovexr-y r= feat y—all+r|lz—all| ||a—alFrom the assumptions, ||a— y|| > ry and also ||y—a|| < r+4r,. Hence the abovery r r, (r+r)+r=r—IVr _— r-———_,|z—al| ||jw—al \lz — alr r 1 a-—lr{ 1—-———_]>r\1——])2r\(l—-—)2r .\|z — all ry a atlThe other case is that ||y — a|| — ||a — al] < 0 in 4.30. Then in this case 4.30 equals(feat (ie all —liy all)||z—al| ||a—alPsIV]a—a l@—yll— (le — all — ly all)