11.10. MAPPINGS NOT ONE TO ONE 337

Theorem 11.9.8 Let U be an open set and let h : U→h(U) be continuous and oneto one and differentiable on the measurable H ⊆U. Then if f ≥ 0 is Lebesgue measurable,∫

h(H)f (y)dmp =

∫H

f (h(x)) |det(Dh(x))|dmp

11.10 Mappings Not One to OneLet H ⊆U and h is differentiable on H. Let Z ≡ {x ∈ H : |detDh(x)|= 0}. Then it ispossible to decompose H \Z into countably many disjoint measurable sets such that h willbe one to one on each of these sets.

Lemma 11.10.1 Let h : H ⊆U ⊆ Rp→ Rp be differentiable on H and let

Z ≡ {x : det(Dh(x)) = 0} .

Then there is a countable set {Fi}i of disjoint Borel sets such that h is one to one on eachof these and [det(Dh(x)) ̸= 0] = H \Z = ∪iFi.

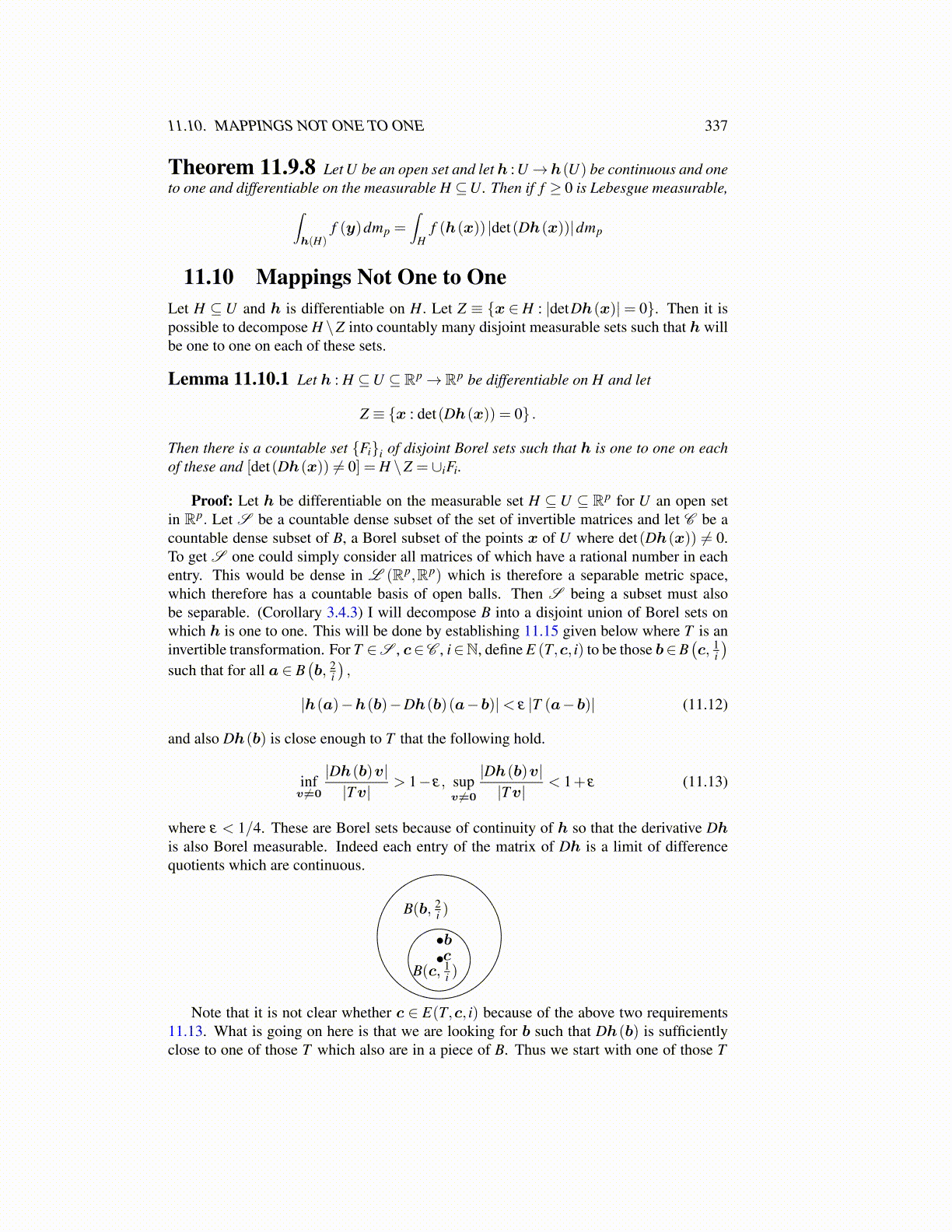
Proof: Let h be differentiable on the measurable set H ⊆U ⊆ Rp for U an open setin Rp. Let S be a countable dense subset of the set of invertible matrices and let C be acountable dense subset of B, a Borel subset of the points x of U where det(Dh(x)) ̸= 0.To get S one could simply consider all matrices of which have a rational number in eachentry. This would be dense in L (Rp,Rp) which is therefore a separable metric space,which therefore has a countable basis of open balls. Then S being a subset must alsobe separable. (Corollary 3.4.3) I will decompose B into a disjoint union of Borel sets onwhich h is one to one. This will be done by establishing 11.15 given below where T is aninvertible transformation. For T ∈S , c∈C , i∈N, define E (T,c, i) to be those b∈B

(c, 1

i

)such that for all a ∈ B

(b, 2

i

),

|h(a)−h(b)−Dh(b)(a−b)|< ε |T (a−b)| (11.12)

and also Dh(b) is close enough to T that the following hold.

infv ̸=0

|Dh(b)v||Tv|

> 1− ε, supv ̸=0

|Dh(b)v||Tv|

< 1+ ε (11.13)

where ε < 1/4. These are Borel sets because of continuity of h so that the derivative Dhis also Borel measurable. Indeed each entry of the matrix of Dh is a limit of differencequotients which are continuous.

•b•c

B(c, 1i )

B(b, 2i )

Note that it is not clear whether c ∈ E(T,c, i) because of the above two requirements11.13. What is going on here is that we are looking for b such that Dh(b) is sufficientlyclose to one of those T which also are in a piece of B. Thus we start with one of those T