16.8. TECHNICAL CONSIDERATIONS 449
F is H p measurable as is E \F because H p (E \F) = 0 from Lemma 16.7.3 and H p
is an outer measure. Recall that sets having outer measure 0 end up being measurablesets. Thus E = F ∪ (E \F) is H p measurable also. This implies from Theorem 16.6.1and Proposition 11.1.2 that if E is Lebesgue measurable, there is Borel F ⊇ E such thatmp (E) = mp (F) = lH p (F) = lH p (E). Now choose β (p) to make the constant l = 1.■
The exact determination of β (p) is more technical.
16.8 Technical ConsiderationsLet α(p) be the volume of the unit ball in Rp. Thus the volume of B(0,r) in Rp is α(p)rp
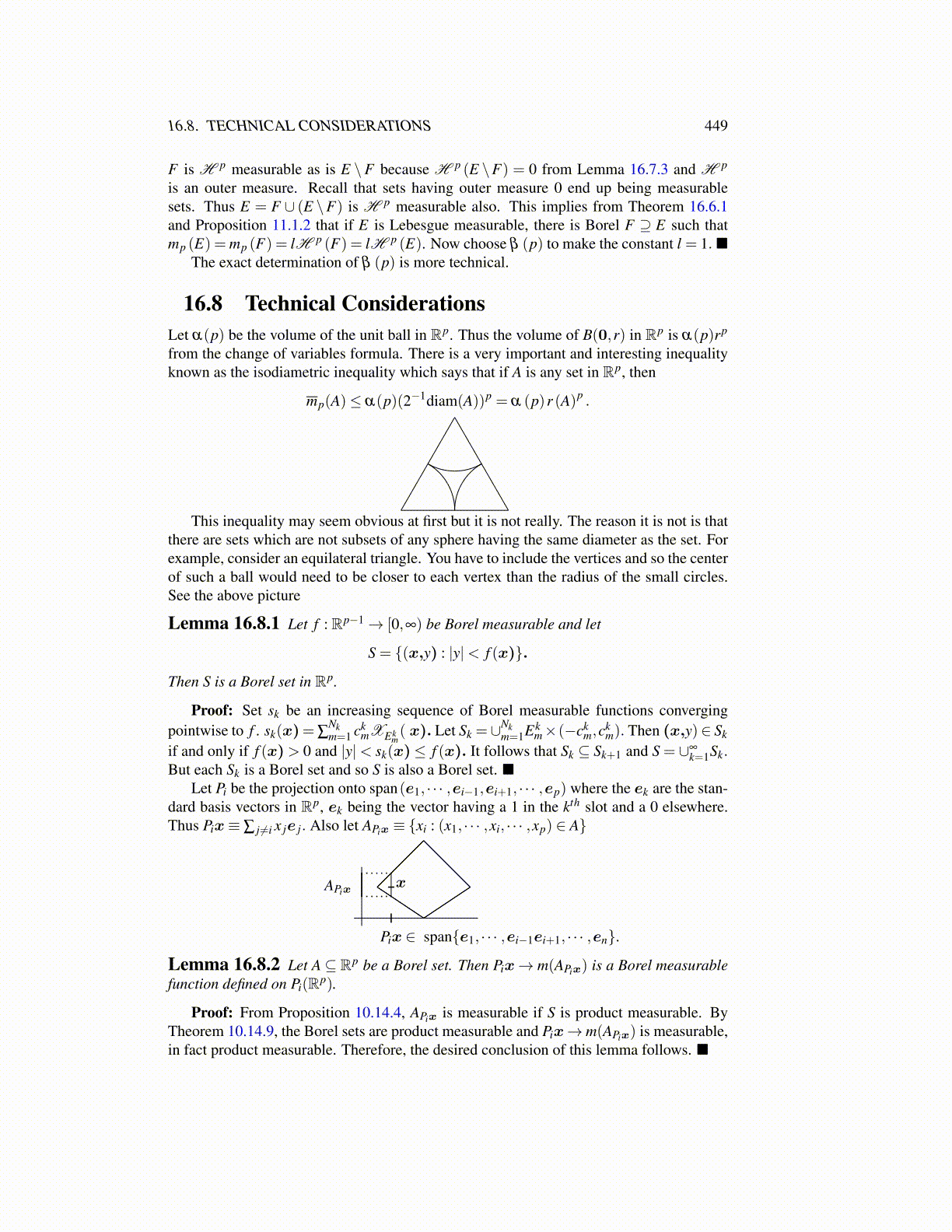
from the change of variables formula. There is a very important and interesting inequalityknown as the isodiametric inequality which says that if A is any set in Rp, then
mp(A)≤ α(p)(2−1diam(A))p = α (p)r (A)p .
This inequality may seem obvious at first but it is not really. The reason it is not is thatthere are sets which are not subsets of any sphere having the same diameter as the set. Forexample, consider an equilateral triangle. You have to include the vertices and so the centerof such a ball would need to be closer to each vertex than the radius of the small circles.See the above picture
Lemma 16.8.1 Let f : Rp−1→ [0,∞) be Borel measurable and let
S = {(x,y) : |y|< f (x)}.
Then S is a Borel set in Rp.
Proof: Set sk be an increasing sequence of Borel measurable functions convergingpointwise to f . sk(x)=∑
Nkm=1 ck
mXEkm( x). Let Sk =∪Nk
m=1Ekm×(−ck
m,ckm). Then (x,y)∈ Sk
if and only if f (x)> 0 and |y|< sk(x)≤ f (x). It follows that Sk ⊆ Sk+1 and S = ∪∞k=1Sk.
But each Sk is a Borel set and so S is also a Borel set. ■Let Pi be the projection onto span(e1, · · · ,ei−1,ei+1, · · · ,ep) where the ek are the stan-
dard basis vectors in Rp, ek being the vector having a 1 in the kth slot and a 0 elsewhere.Thus Pix≡ ∑ j ̸=i x je j. Also let APix ≡ {xi : (x1, · · · ,xi, · · · ,xp) ∈ A}
xAPix
Pix ∈ span{e1, · · · ,ei−1ei+1, · · · ,en}.
Lemma 16.8.2 Let A⊆ Rp be a Borel set. Then Pix→ m(APix) is a Borel measurablefunction defined on Pi(Rp).
Proof: From Proposition 10.14.4, APix is measurable if S is product measurable. ByTheorem 10.14.9, the Borel sets are product measurable and Pix→m(APix) is measurable,in fact product measurable. Therefore, the desired conclusion of this lemma follows. ■