450 CHAPTER 16. HAUSDORFF MEASURE

16.8.1 Steiner Symmetrization

Definition 16.8.3 Define S(A,ei)≡ {x≡ Pix+ yei : |y|< 2−1m(APix)}.

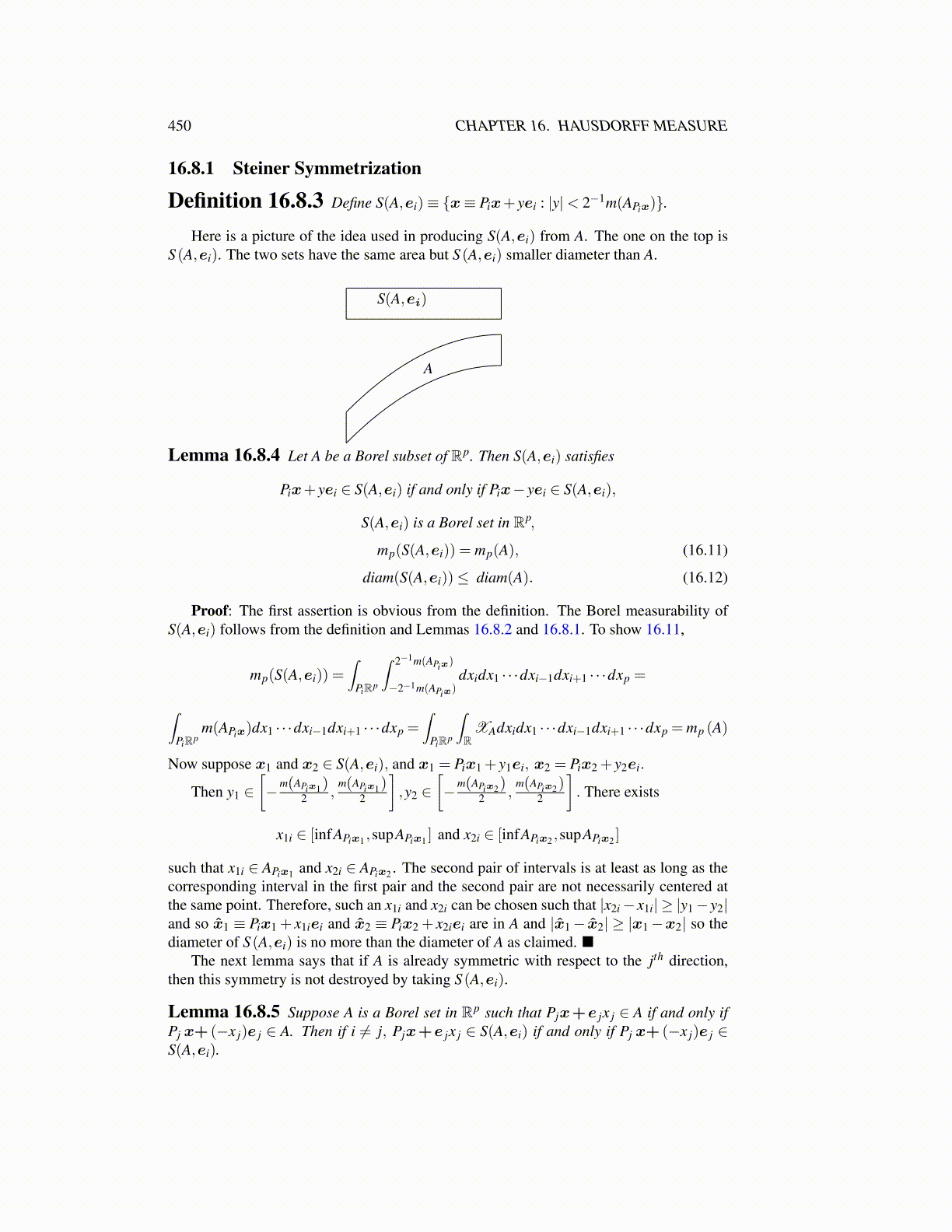
Here is a picture of the idea used in producing S(A,ei) from A. The one on the top isS (A,ei). The two sets have the same area but S (A,ei) smaller diameter than A.

A

S(A,ei)

Lemma 16.8.4 Let A be a Borel subset of Rp. Then S(A,ei) satisfies

Pix+ yei ∈ S(A,ei) if and only if Pix− yei ∈ S(A,ei),

S(A,ei) is a Borel set in Rp,

mp(S(A,ei)) = mp(A), (16.11)

diam(S(A,ei))≤ diam(A). (16.12)

Proof: The first assertion is obvious from the definition. The Borel measurability ofS(A,ei) follows from the definition and Lemmas 16.8.2 and 16.8.1. To show 16.11,

mp(S(A,ei)) =∫

PiRp

∫ 2−1m(APix)

−2−1m(APix)dxidx1 · · ·dxi−1dxi+1 · · ·dxp =

∫PiRp

m(APix)dx1 · · ·dxi−1dxi+1 · · ·dxp =∫

PiRp

∫R

XAdxidx1 · · ·dxi−1dxi+1 · · ·dxp =mp (A)

Now suppose x1 and x2 ∈ S(A,ei), and x1 = Pix1 + y1ei, x2 = Pix2 + y2ei.

Then y1 ∈[−m(APix1)

2 ,m(APix1)

2

],y2 ∈

[−m(APix2)

2 ,m(APix2)

2

]. There exists

x1i ∈ [infAPix1 ,supAPix1 ] and x2i ∈ [infAPix2 ,supAPix2 ]

such that x1i ∈ APix1 and x2i ∈ APix2 . The second pair of intervals is at least as long as thecorresponding interval in the first pair and the second pair are not necessarily centered atthe same point. Therefore, such an x1i and x2i can be chosen such that |x2i− x1i| ≥ |y1− y2|and so x̂1 ≡ Pix1 + x1iei and x̂2 ≡ Pix2 + x2iei are in A and |x̂1− x̂2| ≥ |x1−x2| so thediameter of S (A,ei) is no more than the diameter of A as claimed. ■

The next lemma says that if A is already symmetric with respect to the jth direction,then this symmetry is not destroyed by taking S (A,ei).

Lemma 16.8.5 Suppose A is a Borel set in Rp such that Pjx+e jx j ∈ A if and only ifPj x+ (−x j)e j ∈ A. Then if i ̸= j, Pjx+e jx j ∈ S(A,ei) if and only if Pj x+ (−x j)e j ∈S(A,ei).