2.3. CIRCULAR FUNCTIONS 53
centered at this point has exactly half of it subtended by the line. Thus the radian measureof the angle is π . If we identify the radian measure of each of these angles with the labelused for the angle, it follows that the sum of the measures of the angles of the triangle,α +β + γ equals π .
The following proof of the Pythagorean theorem is due to Euclid a few hundred yearsB.C. A right triangle is one in which one of the angles has radian measure π/2. It is calleda right triangle. Thus if this angle is placed with its vertex at (0,0) its sides subtend an arcof length π/2 on the unit circle, a circle with radius 1. The hypotenuse is by definition theside of the right triangle which is opposite the right angle. From the above observation,both of the other angles in a right triangle have radian measure less than π/2
Theorem 2.3.2 (Pythagoras) In a right triangle the square of the length of the hy-potenuse equals the sum of the squares of the lengths of the other two sides.
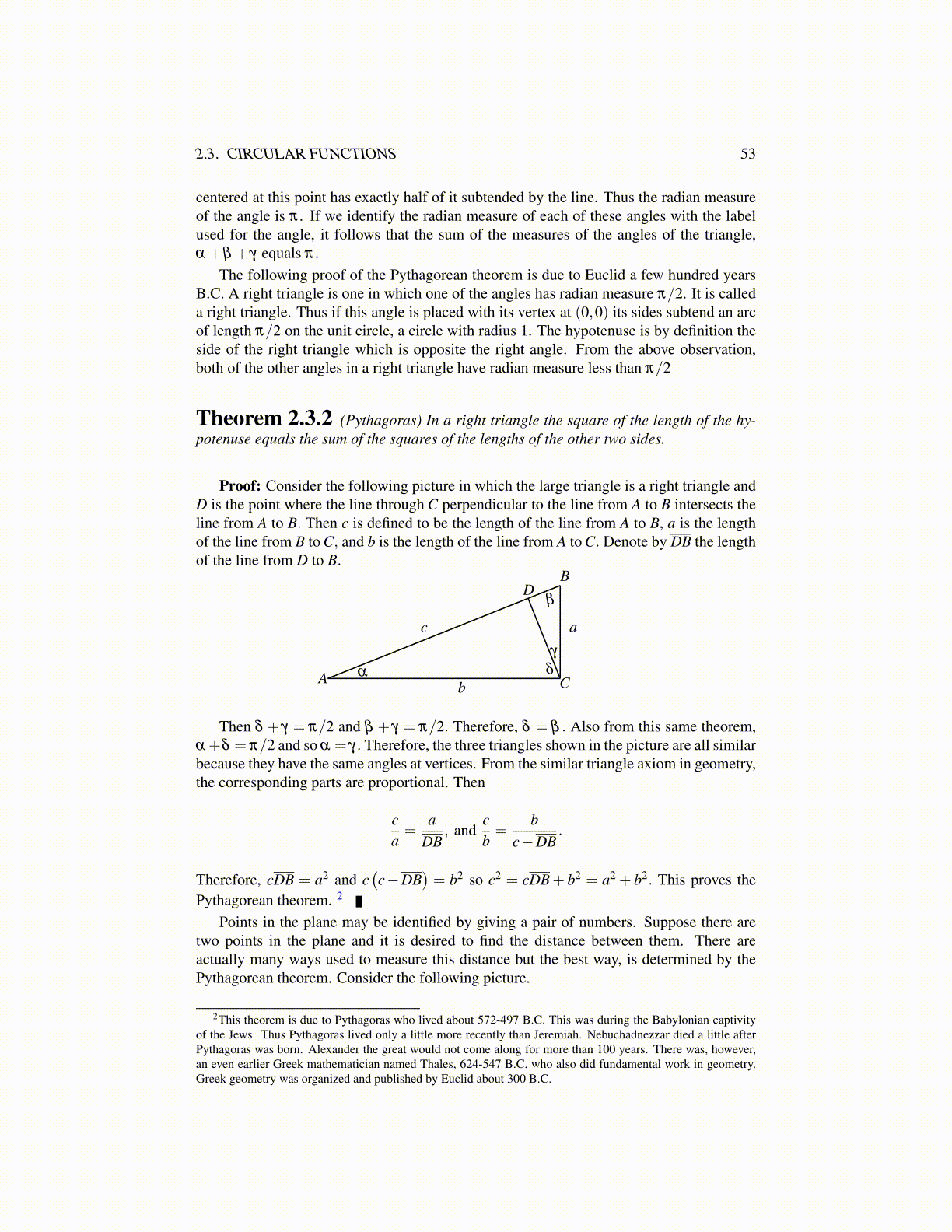
Proof: Consider the following picture in which the large triangle is a right triangle andD is the point where the line through C perpendicular to the line from A to B intersects theline from A to B. Then c is defined to be the length of the line from A to B, a is the lengthof the line from B to C, and b is the length of the line from A to C. Denote by DB the lengthof the line from D to B.
A α
β
γ
δC
B
c a
b
D
Then δ + γ = π/2 and β + γ = π/2. Therefore, δ = β . Also from this same theorem,α+δ = π/2 and so α = γ. Therefore, the three triangles shown in the picture are all similarbecause they have the same angles at vertices. From the similar triangle axiom in geometry,the corresponding parts are proportional. Then
ca=
aDB
, andcb=
bc−DB
.
Therefore, cDB = a2 and c(c−DB
)= b2 so c2 = cDB+ b2 = a2 + b2. This proves the
Pythagorean theorem. 2
Points in the plane may be identified by giving a pair of numbers. Suppose there aretwo points in the plane and it is desired to find the distance between them. There areactually many ways used to measure this distance but the best way, is determined by thePythagorean theorem. Consider the following picture.
2This theorem is due to Pythagoras who lived about 572-497 B.C. This was during the Babylonian captivityof the Jews. Thus Pythagoras lived only a little more recently than Jeremiah. Nebuchadnezzar died a little afterPythagoras was born. Alexander the great would not come along for more than 100 years. There was, however,an even earlier Greek mathematician named Thales, 624-547 B.C. who also did fundamental work in geometry.Greek geometry was organized and published by Euclid about 300 B.C.