2.3. CIRCULAR FUNCTIONS 55
We say that (cos t,sin t) parametrizes the unit circle with respect to arc length, but moreon this much later. The thing to notice here is that a small change in t leads to a smallchange in p(t) and consequently a small change in the x and y coordinates of p(t) whichare defined as cos t and sin t respectively. This is an informal way to say that these functionsof t are continuous, but more about this will be discussed later.
Once you know about the sine and cosine, the other trigonometric functions are definedas follows.
tan(x)≡ sin(x)cos(x)
,cot(x)≡ cos(x)sin(x)
, sec(x)≡ 1cos(x)
, csc(x)≡ 1sin(x)
Of course those which are not the circular functions, sine and cosine, must have a restrictionon their domains because one cannot divide by 0. Since (cos t,sin t) is a point on the unitcircle, it follows that
cos2 t + sin2 t = 1. (2.2)
This is the most fundamental identity in trigonometry. Also, directly from the definition itfollows that
sin(t) =−sin(−t) , cos(t) = cos(−t) (2.3)
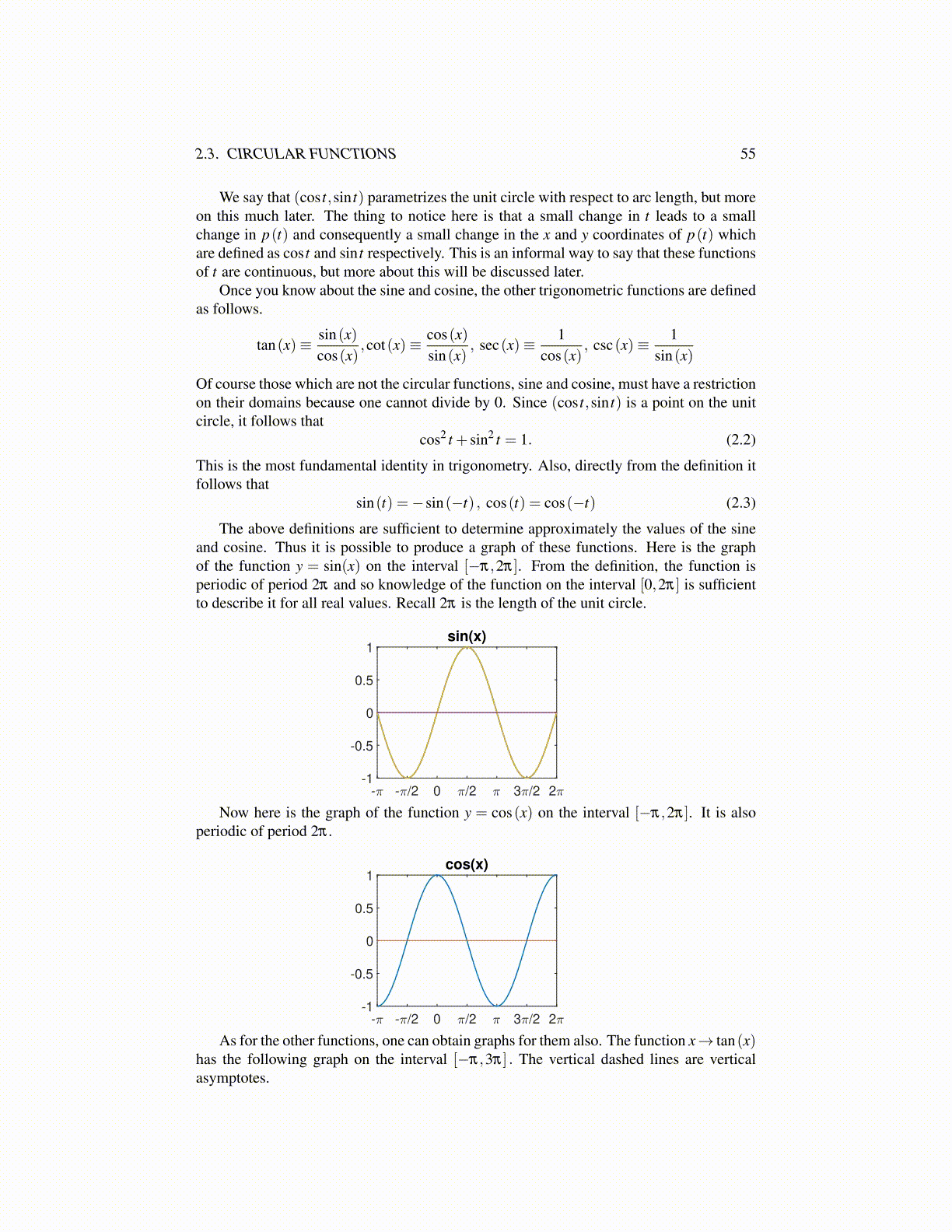
The above definitions are sufficient to determine approximately the values of the sineand cosine. Thus it is possible to produce a graph of these functions. Here is the graphof the function y = sin(x) on the interval [−π,2π]. From the definition, the function isperiodic of period 2π and so knowledge of the function on the interval [0,2π] is sufficientto describe it for all real values. Recall 2π is the length of the unit circle.
- - /2 0 /2 3 /2 2-1
-0.5
0
0.5
1sin(x)
Now here is the graph of the function y = cos(x) on the interval [−π,2π]. It is alsoperiodic of period 2π .
- - /2 0 /2 3 /2 2-1
-0.5
0
0.5
1cos(x)
As for the other functions, one can obtain graphs for them also. The function x→ tan(x)has the following graph on the interval [−π,3π] . The vertical dashed lines are verticalasymptotes.