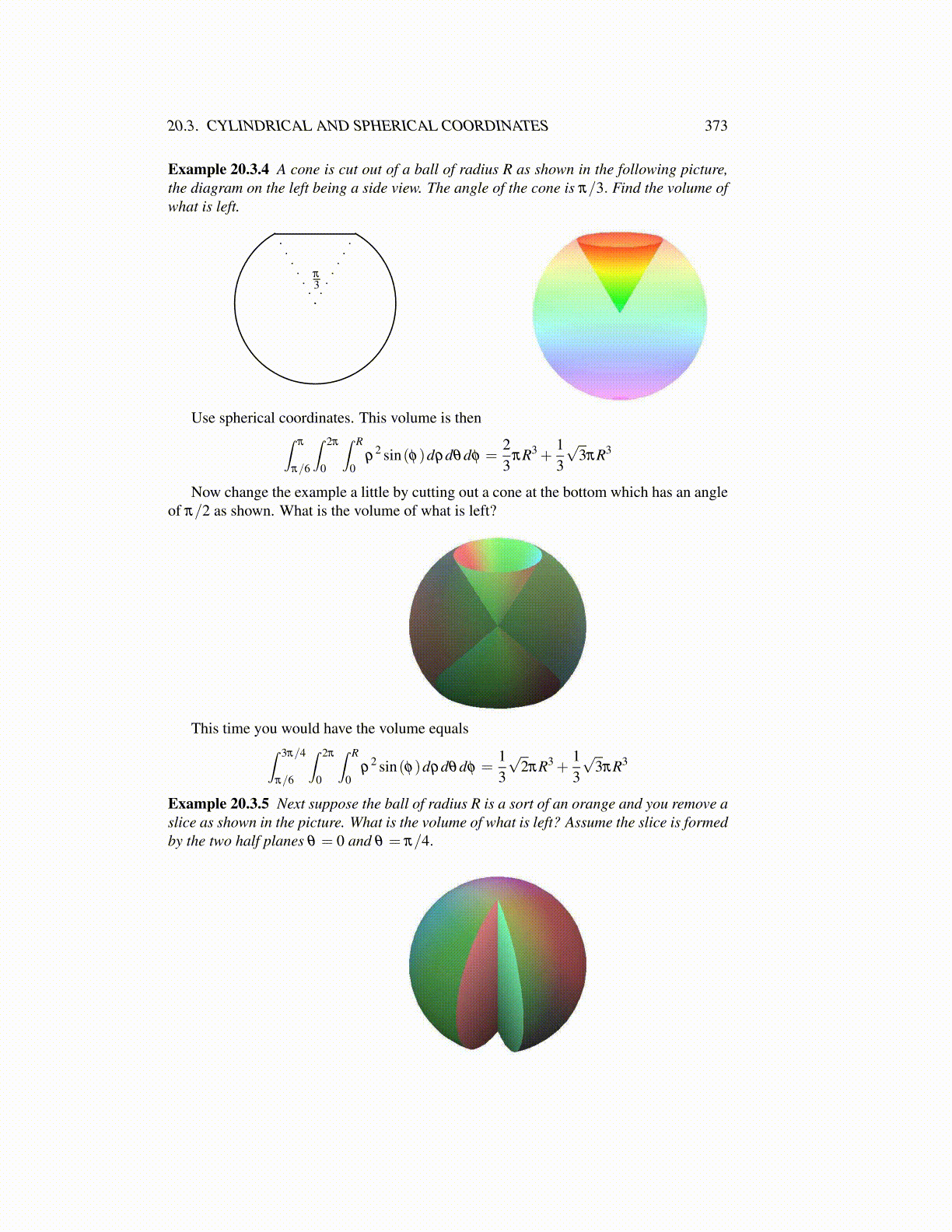
20.3. CYLINDRICAL AND SPHERICAL COORDINATES 373

Example 20.3.4 A cone is cut out of a ball of radius R as shown in the following picture,the diagram on the left being a side view. The angle of the cone is π/3. Find the volume ofwhat is left.

π

3

Use spherical coordinates. This volume is then∫π

π/6

∫ 2π

0

∫ R

0ρ

2 sin(φ)dρdθdφ =23

πR3 +13

√3πR3

Now change the example a little by cutting out a cone at the bottom which has an angleof π/2 as shown. What is the volume of what is left?

This time you would have the volume equals∫ 3π/4

π/6

∫ 2π

0

∫ R

0ρ

2 sin(φ)dρdθdφ =13

√2πR3 +

13

√3πR3

Example 20.3.5 Next suppose the ball of radius R is a sort of an orange and you remove aslice as shown in the picture. What is the volume of what is left? Assume the slice is formedby the two half planes θ = 0 and θ = π/4.