11.3. THE ABSTRACT LEBESGUE INTEGRAL 237
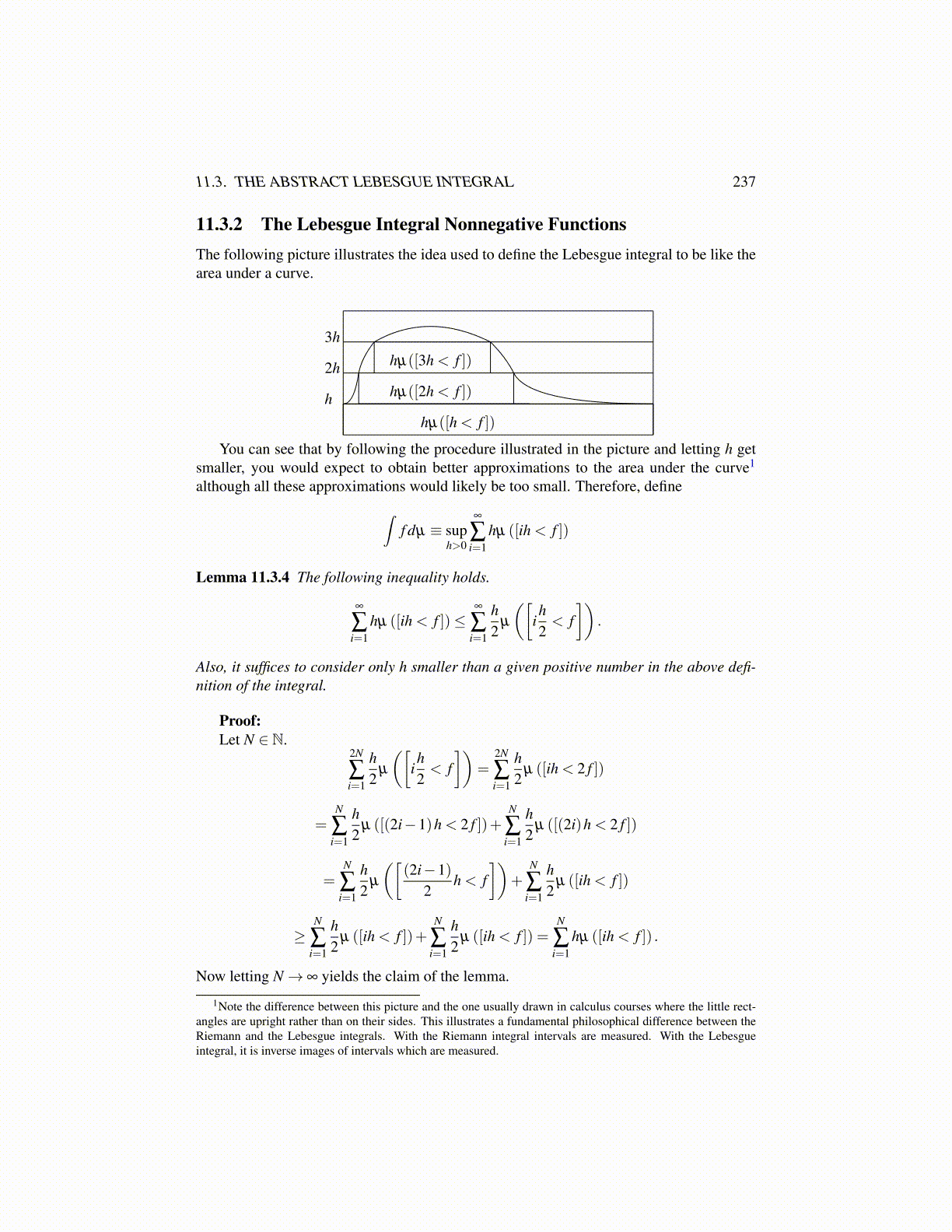
11.3.2 The Lebesgue Integral Nonnegative FunctionsThe following picture illustrates the idea used to define the Lebesgue integral to be like thearea under a curve.
h
2h
3h
hµ([h < f ])
hµ([2h < f ])
hµ([3h < f ])
You can see that by following the procedure illustrated in the picture and letting h getsmaller, you would expect to obtain better approximations to the area under the curve1
although all these approximations would likely be too small. Therefore, define∫f dµ ≡ sup
h>0
∞
∑i=1
hµ ([ih < f ])
Lemma 11.3.4 The following inequality holds.
∞
∑i=1
hµ ([ih < f ])≤∞
∑i=1
h2
µ
([ih2< f])
.
Also, it suffices to consider only h smaller than a given positive number in the above defi-nition of the integral.
Proof:Let N ∈ N.
2N
∑i=1
h2
µ
([ih2< f])
=2N
∑i=1
h2
µ ([ih < 2 f ])
=N
∑i=1
h2
µ ([(2i−1)h < 2 f ])+N
∑i=1
h2
µ ([(2i)h < 2 f ])
=N
∑i=1
h2
µ
([(2i−1)
2h < f
])+
N
∑i=1
h2
µ ([ih < f ])
≥N
∑i=1
h2
µ ([ih < f ])+N
∑i=1
h2
µ ([ih < f ]) =N
∑i=1
hµ ([ih < f ]) .
Now letting N→ ∞ yields the claim of the lemma.
1Note the difference between this picture and the one usually drawn in calculus courses where the little rect-angles are upright rather than on their sides. This illustrates a fundamental philosophical difference between theRiemann and the Lebesgue integrals. With the Riemann integral intervals are measured. With the Lebesgueintegral, it is inverse images of intervals which are measured.