11.3. THE ABSTRACT LEBESGUE INTEGRAL 239

Proof: Consider 11.3.11 first. Without loss of generality, you can assume 0 < a1 <a2 < · · ·< ap and that µ (Ei)< ∞. Let ε > 0 be given and let

δ 1

p

∑i=1

µ (Ei)< ε.

Pick δ < δ 1 such that for h < δ it is also true that

h <12

min(a1,a2−a1,a3−a2, · · · ,an−an−1) .

Then for 0 < h < δ

∞

∑k=1

hµ ([s > kh]) =∞

∑k=1

h∞

∑i=k

µ ([ih < s≤ (i+1)h])

=∞

∑i=1

i

∑k=1

hµ ([ih < s≤ (i+1)h])

=∞

∑i=1

ihµ ([ih < s≤ (i+1)h]) . (11.3.13)

Because of the choice of h there exist positive integers, ik such that i1 < i2 < · · · ,< ip and

i1h < a1 ≤ (i1 +1)h < · · ·< i2h < a2 <

< (i2 +1)h < · · ·< iph < ap ≤ (ip +1)h

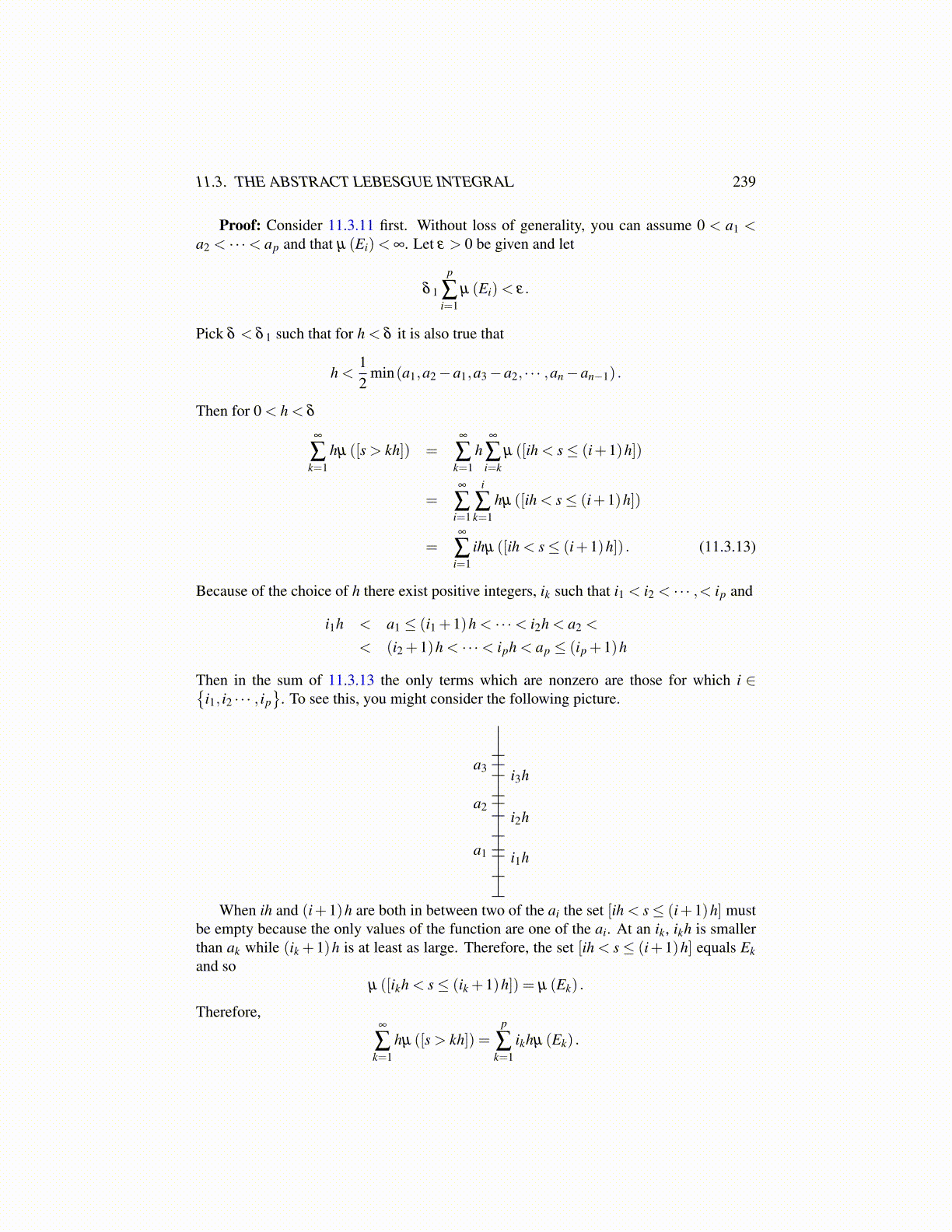
Then in the sum of 11.3.13 the only terms which are nonzero are those for which i ∈{i1, i2 · · · , ip

}. To see this, you might consider the following picture.

a1

a2

a3

i1h

i3h

i2h

When ih and (i+1)h are both in between two of the ai the set [ih < s≤ (i+1)h] mustbe empty because the only values of the function are one of the ai. At an ik, ikh is smallerthan ak while (ik +1)h is at least as large. Therefore, the set [ih < s≤ (i+1)h] equals Ekand so

µ ([ikh < s≤ (ik +1)h]) = µ (Ek) .

Therefore,∞

∑k=1

hµ ([s > kh]) =p

∑k=1

ikhµ (Ek) .