12.9. PRODUCT MEASURES 307
Note that if E = A×B as above, then∫ ∫XE (x,y)dµdν =
∫ ∫XA×B (x,y)dµdν
=∫ ∫
XA (x)XB (y)dµdν = µ (A)ν (B) = ρ (E)
and so there is no contradiction between 12.9.31 and 12.9.30.The first goal is to show that for Q ∈R, 12.9.32 and 12.9.33 both hold. That is, x→
XQ (x,y) is µ measurable for all y and y→∫
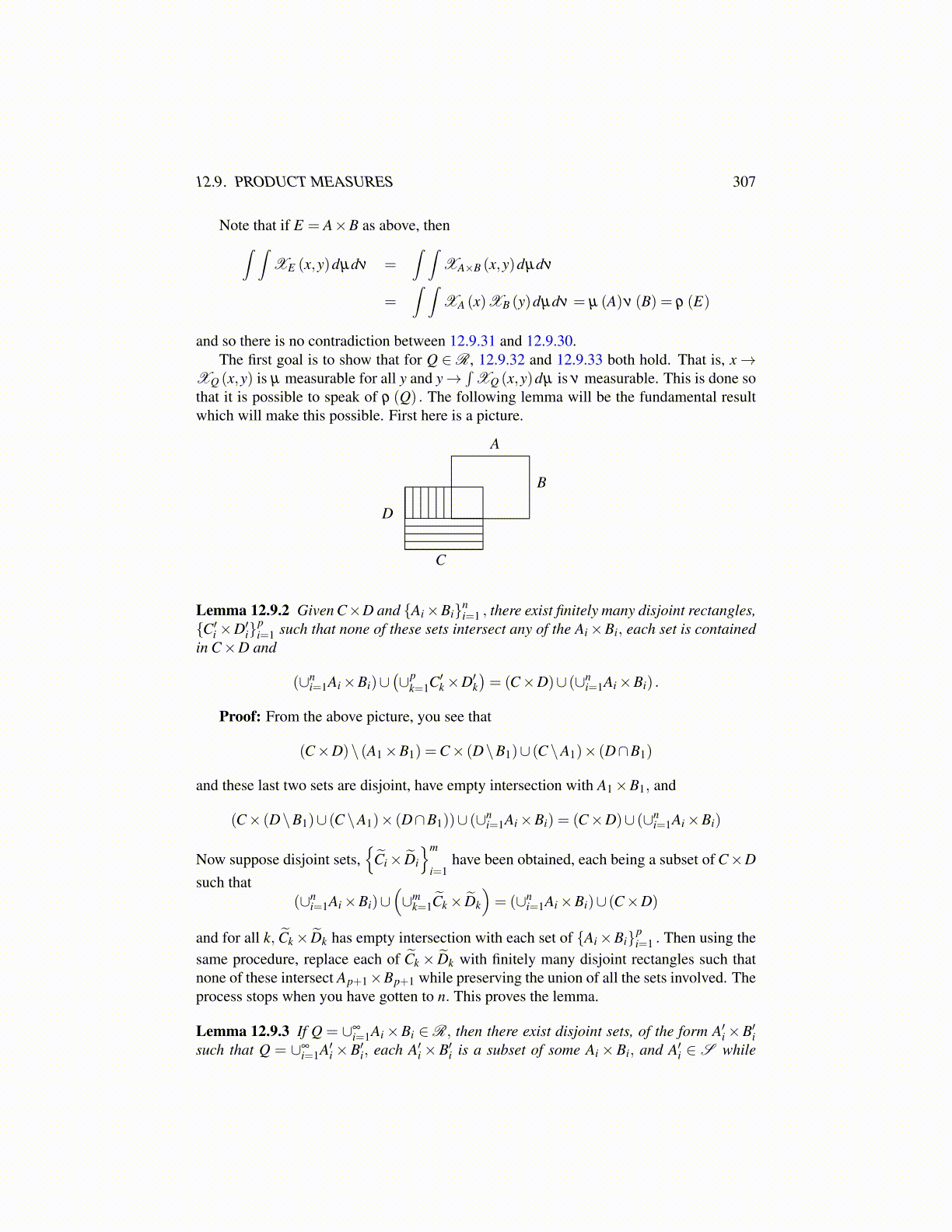
XQ (x,y)dµ is ν measurable. This is done sothat it is possible to speak of ρ (Q) . The following lemma will be the fundamental resultwhich will make this possible. First here is a picture.
C
D
A
B
Lemma 12.9.2 Given C×D and {Ai×Bi}ni=1 , there exist finitely many disjoint rectangles,
{C′i ×D′i}pi=1 such that none of these sets intersect any of the Ai×Bi, each set is contained
in C×D and
(∪ni=1Ai×Bi)∪
(∪p
k=1C′k×D′k)= (C×D)∪ (∪n
i=1Ai×Bi) .
Proof: From the above picture, you see that
(C×D)\ (A1×B1) =C× (D\B1)∪ (C \A1)× (D∩B1)
and these last two sets are disjoint, have empty intersection with A1×B1, and
(C× (D\B1)∪ (C \A1)× (D∩B1))∪ (∪ni=1Ai×Bi) = (C×D)∪ (∪n
i=1Ai×Bi)
Now suppose disjoint sets,{
C̃i× D̃i
}m
i=1have been obtained, each being a subset of C×D
such that(∪n
i=1Ai×Bi)∪(∪m
k=1C̃k× D̃k
)= (∪n
i=1Ai×Bi)∪ (C×D)
and for all k, C̃k× D̃k has empty intersection with each set of {Ai×Bi}pi=1 . Then using the
same procedure, replace each of C̃k× D̃k with finitely many disjoint rectangles such thatnone of these intersect Ap+1×Bp+1 while preserving the union of all the sets involved. Theprocess stops when you have gotten to n. This proves the lemma.
Lemma 12.9.3 If Q = ∪∞i=1Ai×Bi ∈R, then there exist disjoint sets, of the form A′i×B′i
such that Q = ∪∞i=1A′i×B′i, each A′i×B′i is a subset of some Ai×Bi, and A′i ∈ S while