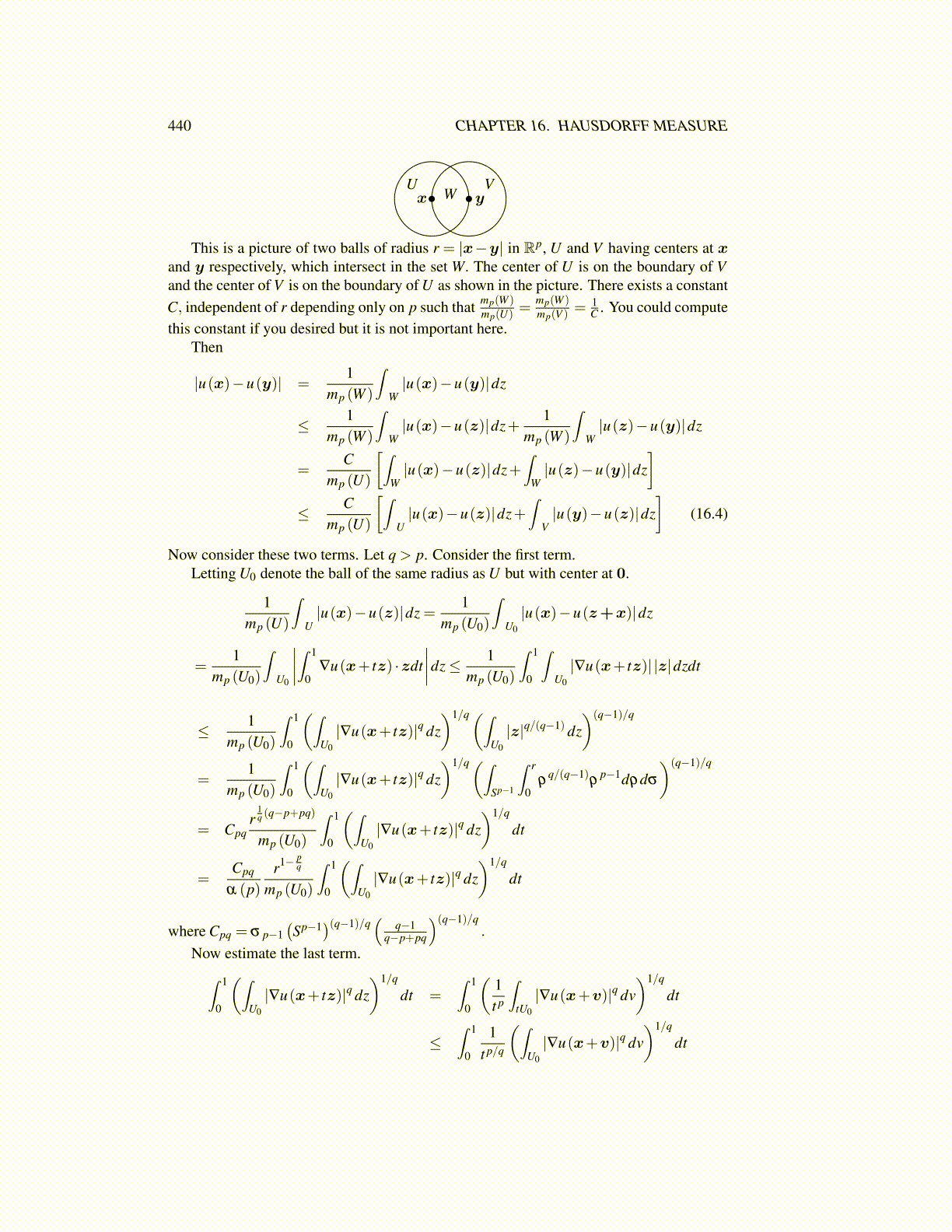
440 CHAPTER 16. HAUSDORFF MEASURE
xU
WVy
This is a picture of two balls of radius r = |x−y| in Rp, U and V having centers at xand y respectively, which intersect in the set W. The center of U is on the boundary of Vand the center of V is on the boundary of U as shown in the picture. There exists a constantC, independent of r depending only on p such that mp(W )
mp(U) =mp(W )mp(V ) =
1C . You could compute
this constant if you desired but it is not important here.Then
|u(x)−u(y)| =1
mp (W )
∫W|u(x)−u(y)|dz
≤ 1mp (W )
∫W|u(x)−u(z)|dz+
1mp (W )
∫W|u(z)−u(y)|dz
=C
mp (U)
[∫W|u(x)−u(z)|dz+
∫W|u(z)−u(y)|dz
]≤ C
mp (U)
[∫U|u(x)−u(z)|dz+
∫V|u(y)−u(z)|dz
](16.4)
Now consider these two terms. Let q > p. Consider the first term.Letting U0 denote the ball of the same radius as U but with center at 0.
1mp (U)
∫U|u(x)−u(z)|dz =
1mp (U0)
∫U0
|u(x)−u(z+x)|dz
=1
mp (U0)
∫U0
∣∣∣∣∫ 1
0∇u(x+ tz) ·zdt
∣∣∣∣dz≤ 1mp (U0)
∫ 1
0
∫U0
|∇u(x+ tz)| |z|dzdt
≤ 1mp (U0)
∫ 1
0
(∫U0
|∇u(x+ tz)|q dz)1/q(∫
U0
|z|q/(q−1) dz)(q−1)/q
=1
mp (U0)
∫ 1
0
(∫U0
|∇u(x+ tz)|q dz)1/q(∫
Sp−1
∫ r
0ρ
q/(q−1)ρ
p−1dρdσ
)(q−1)/q
= Cpqr
1q (q−p+pq)
mp (U0)
∫ 1
0
(∫U0
|∇u(x+ tz)|q dz)1/q
dt
=Cpq
α (p)r1− p
q
mp (U0)
∫ 1
0
(∫U0
|∇u(x+ tz)|q dz)1/q
dt
where Cpq = σ p−1(Sp−1
)(q−1)/q(
q−1q−p+pq
)(q−1)/q.
Now estimate the last term.∫ 1
0
(∫U0
|∇u(x+ tz)|q dz)1/q
dt =∫ 1
0
(1t p
∫tU0
|∇u(x+v)|q dv)1/q
dt
≤∫ 1
0
1t p/q
(∫U0
|∇u(x+v)|q dv)1/q
dt